Moment Identities for Skorohod Integrals on Guichardet-Fock Spaces ()
1. Introduction
The quantum stochastic calculus [1] [2] developed by Hudson and Parthasarathy is essentially a noncommutative extension of classical Ito stochastic calculus. In this theory, annihilation, creation, and number operator processes in boson Fock space play the role of “quantum noises”, [3] which are in continuous time. In 2002, Attal [4] discussed and extended quantum stochastic calculus by means of the Skorohod integral of anticipation processes and the related gradient operator on Guichardet-Fock spaces. Usually, Fock spaces as the models of the Particle Systems are widely used in quantumphysics. Meanwhile, vacuum states described by empty set on Guichardet-Fockspaces play very important role at quantum physics.
Recently Privault [5] [6] developed a Malliavin-type theory of stochastic calculus on Wiener spaces and showed its several interesting applications. In his article, Privault surveyed the moment identities for Skorohod integral on Wiener spaces. It is well known that Guichardet-Fock space F and Wiener space W are Wiener- Ito-Segal isomorphic. Motivated by the above, we would like to study the momentidentities for Skorohod integraon Guichardet-Fock spaces.
This paper is organized as follows. Section 2, we fix some necessarynotations and recall main notions and facts about Skorohod integralin Guichardet-Fock spaces. Section 3 states our main results.
2. Notations
In this section, we fix some necessary notations and recall mainnotions in Guichardet-Fock spaces. For detail formulation of Skorohod integrals, we refer reader to [4].
Let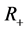 
be the set of all nonnegative real numbers and 
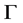the finite power set of
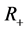, namely
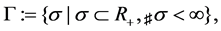
where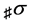 
denotes the cardinality of 
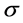as a set. Particularly, let 
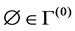be an atom of measure 1. We denote by 
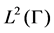the usual space of square integral real-valued functions on
.
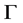Fixing a complex separable Hilbert space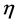
, Guichardet-Fock space tensor product
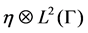, which we identify with the space of square-integrable functions
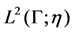, and is denoted by F.
For a Hilbert space-valued map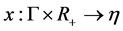
, let
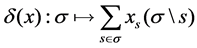
denotes the Skorohod integral operator. For a vector space-valued map![]()
, let ![]()
and ![]()
be the maps ![]()
given by
![]()
respectively denote the stochastic gradient operator of f and the adapted gradient operator of f. Moreover, we write ![]()
for the domain of the stochastic gradient as an unbounded Hilbert apace operator:
![]()
.
Definition 2.1 For the map![]()
, the value of Skorohod integral ![]()
at empty set is called the expectation of ![]()
on Guichardet-Fock space and is denoted by ![]()
i.e.![]()
.
Lemma 2.1 Let x be a map![]()
, if x is square integrable and the function ![]()
is integrable, then ![]()
and
![]()
(2.1)
we denote
![]()
Lemma 2.2 Let ![]()
and let ![]()
be Skorohod integrable, if the map
![]()
is integrable, then
![]()
(2.2)
Lemma 2.3 Let ![]()
be measurable. For![]()
, we have
![]()
(2.3)
where![]()
,![]()
.
Proof In view of the identity
![]()
we have
![]()
3. Moment Identities for Skorohod Integrals
Theorem 3.1 For any ![]()
and![]()
, we have
![]()
(3.1)
where
![]()
For ![]()
the above identity coincides with (2.1).
We will need the following lemma.
Lemma 3.1 Let ![]()
and![]()
. Then for all ![]()
we have
![]()
Proof Using relation (2.2), (2.3), we obtain
![]()
and
![]()
Proof of Theorem 3.1, We decompose
![]()
then we apply lemma 3.1, which yields (3.1).
Acknowledgements
The authors are extremely grateful to the referees for their valuable comments and suggestions on improvement of the first version of the present paper. The authors are supported by National Natural Science Foundation of China (No. 11261027 and No. 11461061), supported by scientific research projects in Colleges and Universities in Gansu province (No. 2015A-122) and supported by Doctoral research start-up fund project of Lanzhou City Universities (No. LZCU-BS2015-02) and SRPNWNU (No. NWNU-LKQW-14-2).