The Impact of the Earth’s Movement through the Space on Measuring the Velocity of Light

Miloš Čojanović

Independent Researcher, Montreal, Canada.

DOI: 10.4236/jamp.2016.46121                

Abstract

Goal of this experiment is basically measuring the velocity of light. As usual we will measure two-way velocity of light (from A to B and back). In contrast to the similar experiments we will not assume that speeds of light from A to B and from B to A are equal. To achieve this we will take into account Earth’s movement through the space, rotation around its axis and apply “least squares method for cosine function”, which will be explained in Section 9. Assuming that direction East-West is already known, one clock, a source of light and a mirror, is all equipment we need for this experiment.

Keywords

Speed of Light, One Way Speed of Light, Least Squares Method for Cosine Function

Received 16 April 2016; accepted 26 June 2016; published 29 June 2016

1. Introduction

Observe the planet Earth. The Earth orbits the Sun. For this motion we will join the vector v₁. Sun orbits the center of the Milky Way. For this motion we will join the vector v₂. In relation to the center of the Milky Way, we can join to the Earth movement sum of vectors

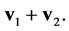
It is also known that our Galaxy is moving relative to other galaxies (or to a point in the space outside the Milky Way Galaxy). Similarly, to this motion we could join the vector v₃.

Denote by v the sum of all these vectors

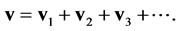(1)

At the end of the sum three points are left, because eventually there may be some other movements.

In the period of 24 h vectors v₂, v₃ can be taken as constants, while the vector v₁ by making a certain error could also be taken as constant.

Thus for the Earth’s motion through the space within 24 h, we can join the constant vector v.

The speed and direction Earth orbits the Sun are known, and let v₀ represent its avarage speed.

Suppose that some approximate values for vectors v₂ and v₃ are known as well. On the basis of these values, let suppose that we have inequality

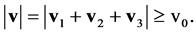(2)

2. Planning an Experiment

Suppose that an arbitrary point A is given. Earth rotation axis will be taken as the z coordinate, and as the plane xy we will take the plane passing through point A and perpendicular to the z axis. In this case it is natural to take section of the plane xy and z axis as the center of the coordinate system. In addition to point A let the points B and D are given. Line AB lies in the plane xy and parallel to the direction of the Earth’s rotation. Distance AB will be marked with L. For the x axis, at some initial time t₀, we will take the line in the plane xy, parallel to AB. The projection of the vector v in the plane xy denote by v_xy. Due to the Earth’s rotation the direction of AB will be changed, so that it will be changed the angle, marked by Φ, between the x axis (which remained fixed) and the line AB. Let at point A we have a clock and some source of light. Suppose that speed of light in the direction AB is given by equation

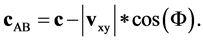(1)

Point D will be chosen so the line AD is parralel to direction South-North. Distance AD is marked by L₁. Angle between line AD and z axis we will denote by j. Angle j actually represents Latitude of point A on the Earth’s surface, thus it remains unchanged during the experiment.

The projection of the vector v on z axis denote by v_z (actually v₂ + v₃, because v₁ is perpendicular on z axis). Assume that the speed of signal in the direction AD is given by equation

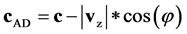(2)

where c represents “velocity of light in vacuum for a body at rest”. Our aim is to find the constant c, vectors v_xy and v_z.

3. Conducting an Experiment

In some moment T₀ we will send signal from point A to point B. The angle between the axis x and v_xy is marked by Θ.

Once the signal arrived at point B it will be reflected back to point A.

Difference between the time when the signal was being sent from point A, and the time when the signal reached to the point A is denoted by t₀.

At the same time we will send signal from point A to D and return back to point A. Difference between the time when signal was being sent and reached to point A we will denote by t₀.

The same procedure will be within 24 h repeated N (N > 4) times, whereas the time between the two sets of consecutive procedure to be same and equal to 24 h/N.

In that way we will get the series {t_i} and {t_i}

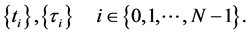(1)

To the each t_i we can join an angle a_i between x axis and line AB.

In that way we get the series

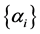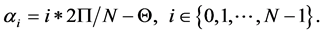where (2)

By assumption (3.1) the speed of the signal c_i in the direction AB is equal to

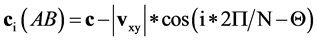(3)

and in opposite direction BA

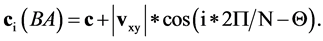(4)

It follows that

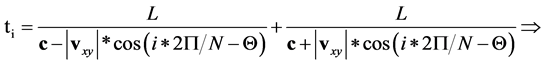(5)

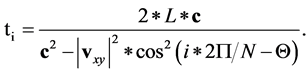(6)

If we swap the roles of the points A and B, we would get the same formula as in (6). Therefore it is completely irrelevant whether direction of the vector v_xy is equal to direction AB or BA.

We assume that

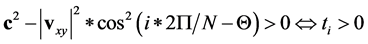
for

It would be in principle our experiment.

4. Computing the Values of c, and Q

In this section we will deal only with the measurements in direction East-West.

Let t_i is given by (3.6) and

(1)

denote the average speed c_i (from point A to point B and back to A).

It follows that can be written as

(2)

where e_i represents some experimental error. Replacing

we get

(3)

in short form

(4)

(5)

, where (6)

The coefficients A, B and Q will be chosen so the sum of squares

(7)

has a minimum value.

To acheive our goal we are going to apply Theorem 1 for k = 2.

For the sake of simplicity we’ve only considered cases when

Thus we have

(8)

(9)

(10)

We’ll make a small digression. From Lemma 1 it follows

In the similiar way we can get

Generally we have. From (9)

(11)

Function Atan () takes values at interval (−P/2, P/2).

If we consider A₀ as function of

From (6) it folows that between the values Θ₁ and Θ₂ we have to choose that one for which A₀ > 0.

From (5) and (6) we can derive values for c and.

(12)

(13)

We don’t know exact direction of vector v_xy, thus positive and negative value are assigned to.

5. Comparison between Two Methods

In this section we will make comparison between “the least squares method” and “the least squares method for cosine function”.

Let consider given by (4.1) as the series of mutually independent measurements.

Let c_m represents the mean value of serial.

(1)

If we apply Least squares method, Variance V₁ is given by

(2)

and standard deviation s₁ by

(3)

Suppose that to the each c_i we joined the time when measurement took place, or rather the angle between the direction of AB and vector v_xy. Expected value E₂(a_i) for “The Least squares method for cosine function” is given by

(4)

where

(5)

Denote a_i by

Let us find Variance V₂ for this method

(6)

(7)

Standard deviation s₂ for this method is given by

(8)

From (7) From (7)

If standard deviation s₂ is bigger then some expected value it means either our measurement are not accurate enough or our method (curve) doesn’t suit to our data.

6. Analysys of South-North Measurements

In this chapter we will deal with the series given by (3.1).

Just to remind that t_i represents time it takes for signal to travel from A to D and back to A in direction South- North.

(1)

(2)

Let

(3)

denote the average speed g_i. In that way we get the series {g_i}

(4)

where e_i represents some experimental error.

Since angle j kept constant value during the experiment we could apply Least squares method to the series given by (4).

Let denote g_m by

(5)

mean value of the series {g_i}.

We can calculate Variance V₁

(6)

and standard deviation s₁

(7)

If standard deviation s₁ is bigger then some expected value we should declare the experiment failed.

Combining equations (4) and (5) we get

(8)

We don’t know exact direction of vector v_z, thus positive and negative value were assigned to.

7. Conclusions

From (5.13) and (7.8) it follows that length of vector v is given by

(1)

while vector v is given by

(2)

Recall (from 2.1) that vector v can be written also as

(3)

Suppose that during one year the same experiments have been repeated 2*K times. In that way we will get the series

(4)

where represents length of vector given by Equation (2) or (3) at i-th try.

Let and denote velocity at which Earth orbits the Sun at -th and i-th try.

Suppose also that origins of vectors and lay on the diameter of Earth orbit around the Sun, so they are parallel but in oposite directions.

Mean value of the serial (3) is given by

(5)

Depending on we will consider following cases:

1)

In other words is significantly less than what is in contradiction to our hypotesis (2.2).

In this case we have to reject hypothesis given by (3.1) and declare that velocity of light is not effected by Earth’s movement through the space.

This results is consistent with some other experiments, for example with Michelson-Morley experiment.

2)

During the experiments in period of one year v₁ is changing, while v₂ + v₃ is keeping the constant value.

Recall that vector v₁ is perpendicular to z axis.

Denote vector u by

(6)

(7)

(8)

(9)

(10)

If we replace and by

(represents average speed Earth orbits the Sun).

From (9) and (10) we can get approximate value for

(11)

We can form serial

(12)

Mean value of the serial (12) is given by

(13)

Let find standard deviation s₁ for serial (13).

If s₁ is bigger then some expected value we have to decline our hypothesis (2.1) and declare the experiment failed.

(14)

(15)

where

For serial (15) mean value u_z is given by

Let standard deviation for serial (15) is marked by s₂.

If s₂ is bigger then some expected value we have to decline our hypothesis (2.1) and declare the experiment failed.

Otherwise hypothesis given by (3.1) holds and we can conclude that velocity of light depends on Earth’s movement through space. In other words velocity of light depends on the direction in which has been measured, what would be in contradiction with Michelson-Morley experiment [1] .

The speed that Solar system moves in the space in this case is given by equation

(16)

Note that while performing the experiment we committed some mistakes.

It was not taken into account the speed of Earth’s rotation. This problem can be solved by conducting an experiment at place closer to the Earth’s poles, and thus the speed of Earth’s rotation taken as small as we want. On other hand this would be counter-productive to our conditions for South-North measurement. Ideally, E-W experiment should be performed on the North/South Pole and S-N experiment at some place on equator.

In addition, within 24 h the Earth changes its direction and the speed at which it revolves around the Sun. We can’t solve this problem but we can assume that this speed is relatively small comparing to total speed at which Earth moves through the space.

8. Lemma 1

If N, k are natural numbers (1 < N, 0< k < N) and Q an arbitrary angle then

(1)

(2)

Proof.

where

Q.E.D.

9. Theorem 1. Least Squares Method for Cosine Function

Suppose we are given the series {c_i}, c_i > 0, and there are at least two p, q thus c_p <> c_q

Let take arbitrary coefficients B, A, Q and form equations

, (1)

Define function g(B, A, Q) by

(2)

We will prove that in case, function g() has a minimum value at point

(3)

(4)

(5)

where, ,.

Proof.

Let B, A and Q have arbitrary values

thus we get

(6)

In that way we can reduce function g() from function of three variables to fuction of two variables A and Q, keeping coefficent B fixed and equal to c_m.

Now we can write the function g() in the form

(7)

In order to find minimum for function g(), first we have to find partial derivates with respect to A and Q and critical point (A₀, Q₀)

(8)

Let us find the first partial derivatives

(9)

(10)

1)

In this case we would have

It’s easy to prove that g() has minimum at

2)

(11)

(12)

Let us look at the Equations (10) and (12)

For we will consider three cases:

1)

From (12) it follows A = 0. We will reject this posibility because.

2)

From (10) it follows

3)

From (10)

From (12)

Now we have to find the second order partial derivatives of g() with respect to A and Q.

(13)

(14)

(15)

(16)

(17)

(18)

Equations given by (13) and (18) are sufficient conditions for minimum.

Q.E.D.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Ditchburn, R.W. (1991) Light. Dover Publications Inc., New York.%%%