Effect of Hall Currents and Variable Fluid Properties on MHD Flow past Stretching Vertical Plate by the Presence of Radiation

Khaled K. Jaber

Department of Mathematics, Faculty of Science and Information Technology, Zarqa University, Zarqa, Jordan.

DOI: 10.4236/jamp.2014.29101                

Abstract

This paper investigates the effects of Hall currents and radiation on free-convective steady laminar boundary-layer flow past a semi-infinite vertical plate for large temperature differences. A uniform magnetic field is applied perpendicular to the plate. The fluid density is assumed to vary exponentially and the thermal conducting linearly with temperature. The fluid viscosity is assumed to vary as a reciprocal of a linear function of temperature. The usual Boussinesq approximation is neglected. The nonlinear boundary layer equations governing the problem under consideration are solved numerically by applying an efficient numerical technique based on the shooting method. The effects of the magnetic parameter , the Hall parameter m, the density/temperature parameter n, the radiation parameter N , the thermal conductivity parameter S, the viscosity temperature , and the temperature ratio parameter are examined on the velocity and temperature distribution as well as the coefficient of heat flux and shearing stress at the plate.

Keywords

MHD, Hall Currents, Radiation, Variable Fluid Properties

1. Introduction

The study of natural convection boundary layer flow of an electrically conducting fluid over a continuously stretching heated semi-infinite plate is considered very essential to understand the behavior of the performance of fluid motion in several applications. This is because it serves understanding of some phenomenon occurring in several environmental and engineering fields. Prominent applications are the aerodynamic extrusion of plastic sheets, cooling of an infinite metallic plate in a cooling path, Fibers spinning and continuous casting, glass blowing, packed bed reactors or absorbent and others. The analysis of such flow forms the bases of a series of further investigations for laminar boundary layers. The first who presented boundary layer flow over a continuous solid surface with constant speed is Sakiadis [1] [2] . Erickson et al. [3] extended Sakiadis problem to include blowing or suction at the moving plate and study its effects on heat and mass transfer in the boundary layer. Vayjravelu and Hadyinicolaou [4] studied the convective heat transfer in an electrically conducting fluid near an isothermal stretching sheet with uniform free stream. Various aspects of this problem have been studied by Grffith [5] , Ghin [6] , Gupta et al. [7] and Gorla [8] . Many investigations have concentrated on the problem of a stretched sheet with a linear velocity and different thermal boundary conditions, see for instance, Chakrabarti et al. [9] , Rajagopal et al. [10] and Chamkha [11] . The problem becomes more interesting when the viscous and thermal boundary layer is subjected to the action of an applied magnetic filed. Free-convection flow with mass transfer along a vertical plate in the presence of magnetic field has been investigated by Elbashbeshy [12] . another problem in this filed is the study of Hall current effects on the consequent flow and heat transfer characteristics that are brought about by the movement of a stretched isothermal sheet in the presence of a strong magnetic field. The effect of Hall current on unsteady hydromagnetic free convective flow past an infinite heated vertical plate is studied by Abo-Eldahab et al. [13] and Khaled K. Jaber [14] . Pop and Watanabe [15] studied the Hall effects on the steady boundary layer free convection flow about a semi-infinite vertical flat plate. Recently, Abo-Eldahab [16] studied the Hall effect on MHD free-convection flow past a stretching surface with uniform free stream. Also, Khaled K. Jaber [17] studied the Hall and ion slip currents on MHD free-convective heat generating flow past a semi-infinite vertical flat plate. Joule heating effect on MHD fee-convective flow of a micropolar fluid is studied by Abd El-Hakiem et al. [18] .

Most of the effort in understanding fluid radiation is devoted to the derivation of reasonable simplifications. One of these simplifications was made by Cogley et al. [3] who assumed that the fluid was in the optical thin limit and, accordingly the fluid did not absorb its own radiation but it only absorbed radiation emitted by the boundaries.

Accordingly, Cogley et al. showed that for an optically thin nongray gas near equilibrium, the following relation holds:

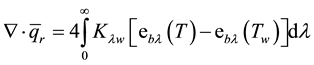(1)

In addition, they simplified (1) by assuming small temperature differences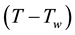. Hence, under this assumption, (1) reduces to

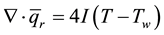
where

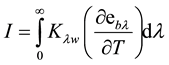(2)

For an optically thick gas, the gas self-absorption rises and the situation becomes difficult. However, the problem can be simplified by using the Rosseland approximation [19] , which relates the radiative heat flux to the gradient of the total emissive power of the gray gas as follows,

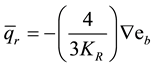(3)

Previous studies of convective flow along vertical plates in the presence of radiation were restricted, in general, to the case where the temperature difference between the plate and the fluid was small. In this case, the fluid’s physical properties such as its viscosity and thermal conductivity may be taken as constant. Also, for small temperature differences, the Boussinesq approximation [20] can be used to treat the fluid density as a constant in the continuity equation, energy equation, and convective terms in the momentum equation and treat it as a variable only in the buoyancy term of the momentum equation.

In situations where there is large temperature differences between the plate and the fluid, the fluid’s physical properties are affected by the high temperature and they can no longer be regarded as constant. Also, in this case, the Boussinesq approximation can no longer be used.

Some recent studies for radiating fluids have taken into account variations of the physical properties with temperature. For example, Aboeldahab [21] studied radiation and variable density effects on the free convective flow of a gas past a semi-infinite vertical plate and showed that for high-temperature differences the Boussinesq approximation leads to substantial errors in velocity and temperature distributions also Jaber [22] studied the combined effects of Hall currents and variable Viscosity on Non-Newtonian MHD flow past a stretching vertical plate. He showed that the variable viscosity effect the temperature and flow velocity. Aboledahab and El Gendy [23] studied the radiation effect on convective heat transfer in an electrically conducting fluid at a stretching surface with variable viscosity and uniform free stream. They showed that the flow characteristics are markedly affected by the variation of viscosity with temperature. Aboeldahab and Salem [24] studied the radiation effect on the MHD free-convective flow of a gas past a semi-infinite vertical plate with variable viscosity. Also, they showed that the flow characteristics are markedly affected by the variation in viscosity with temperature.

Previous studies of convective flow along vertical plates in the presence of radiation were restricted, in general, to the case where the temperature difference between the plate and the fluid was small. In this case, the fluid’s physical properties such as its viscosity and thermal conductivity may be taken as constant. Also, for small temperature differences, the Boussinesq approximation [20] can be used to treat the fluid density as a constant in the continuity equation, energy equation, and convective terms in the momentum equation and treat it as a variable only in the buoyancy term of the momentum equation.

It is worth mentioning that using the Cogley-Vincenti-Gilles model (2) depends on the assumption that the temperature differences are small. Of course, this assumption will lead to an error when the variable property problems (high-temperature differences problems) take place. To avoid such an error we should use (1), which includes a difficult integration. This integration can be simplified by assuming that the gas is gray and so the absorption coefficient is independent of the wavelength (see [15] ). Accordingly, for an optically thin gray gas and for high-temperature differences Equation (1) reduces to

(4)

The above relation is more suitable for expressing the radiation term in the energy equation for the variable physical property problems.

Hence, in the present work, we study Hall currents effects on the MHD free-convective flow of an optically thin gray gas past a semi-infinite vertical plate with variable density, viscosity and thermal conductivity for high temperature differences neglecting the Boussinesq approximation. The nonlinear boundary layer equations, governing the problem, are solved numerically by applying an efficient numerical technique based on the shooting method. The velocity and temperature distributions as well as the coefficient of heat flux and the shearing stress at the plate are determined for different values of the Hall parameter m, the temperature ratio parameter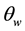, the thermal conductivity parameter, the viscosity-temperature parameter, the magnetic field, and the radiation parameter.

2. Mathematical Formulation

A steady laminar free-convective flow of a viscous gray gas in the optically thin limit past an isothermal semi- infinite vertical plate is considered. The -axis is chosen along the plate and the?axis is taken as normal to it (see Figure 1).

A uniform magnetic field is applied transversely to the direction of the flow. The magnetic Reynolds number is taken to be small enough so that the induced magnetic field can be neglected.

Figure 1. Physical coordinate system.

The viscous dissipation; the radiative heat flux in the -direction, in comparison to the -direction; and the velocity of the gas far away from the plate are assumed to be negligible.

The density is assumed to vary exponentially with temperature as follows: [19]

(5)

where

(6)

The fluid thermal conductivity is assumed to vary as a linear function of temperature in the form

(7)

where is a constant depending on the nature of the fluid. In general, for fluids such as water and air, while for fluids such as lubricating oils.

The fluid viscosity is assumed to vary as a reciprocal of a linear function of temperature in the form (see Lai and Kulacki, ref. [13] )

(8)

or

(9)

where

and are constants and their values depend on the reference state and the thermal property of the fluid. In general for liquids and for gases.

Then the steady laminar two-dimensional free-convective flow is governed by the following boundary-layer equations:

(10)

(11)

(12)

(13)

The physical problem suggests the following initial and boundary condition

(14)

By using Equations (1), (3) and (5), Equations (7), (8) and (9) become

(15)

(16)

(17)

Introducing the following dimensionless variables

(18)

The continuity equation is satisfied by

(19)

From (14) and (15) we find that

(20)

Also, let

(21)

Using the above transformation the governing equations are reduced to:

(22)

(23)

(24)

The boundary conditions are transformed into

(25)

where

And primes denote differentiation with respect to only

The most important characteristics of the flow are shearing stress at the plate

(26)

(27)

And the rate of heat transfer at the plate (Nusselt number)

(28)

3. Results and Discussion

Equations (22), (23) and (24) with the boundary conditions (25), are approximated by a system of nonlinear ordinary differential equations replacing the derivatives with respect to. By two-point backward finite differences with step-size this system is solved numerically by using the fourth-order Runge-Kutta method algorithm with a systematic estimation of, and by the shooting technique to obtain, and.

The value of at infinity is fixed at 2; the requirement that the variation of velocity and temperature distribution is less that 10⁻⁹ between any two successive iteration is employed as the criterion of convergence. We use the symbolic computational software Mathematica to solve this system. Solutions are obtained for the Prandtl number and the Grashoff number.

In view of Equation (18) Equation (5) can be written in the form

(29)

Since varies from 0, at the edge of the boundary layer, to 1 at the vertical plate surface, the density of the fluid adjacent to the plate is related to its free-stream value by the following expression:

From this expression it is obvious that, since free-convection flow is studied, can not be identically zero, otherwise, hence for our problem we have the constant.

It is worth mentioning that when the temperature difference is small, Equation (29) reduces to:

,

where the higher order terms are omitted, in addition, it is assumed that, i.e.

Then according to the above relation the density can be treated as a constant in the continuity equation, energy equation and convective terms in the momentum equation and treated as a variable only in the buoyancy term of the momentum equation (Boussinesq approximation). Therefore, when, Equations (10)-(13) reduce to the Boussinesq equations and for high temperature differences the condition is disregarded.

It is to be noted that, as becomes closer to zero the density variation is negligible except in the buoyancy term as takes values considerably higher than zero the density variation becomes increasingly significant. So according to the definition of the density-temperature parameter, , for a given, variation of means, in fact variation of the temperature difference.

Figure 2 and Figure 3 show the effect of the magnetic field parameter on the velocity and temperature profiles within the boundary layer. In which the increasing of the magnetic field parameter is to decrease the dimensionless primary flow velocity and increases the dimensionless secondary flow velocity. The decreasing of due to the increasing of the Lorentz force, which opposes the flow. The increasing of Hall parameter m increases the secondary flow velocity as shown in Figure 4. Form Figures 5-7 it is observed that the dimensionless velocities and g increase while the dimensionless temperature decreases as the density -temperature parameter increases. An increase in the density temperature parameter means an increase of the velocity in the fluid particles due to an increase in the buoyancy forces (the density variation with temperature increases). Hence as n increases the fluid will be under two forces: the first force increase the velocityies of the fluid due to the increase in the buoyancy forces and the second force decrease the velocities of the fluid due to the decrease in the temperature.

Figure 8 presents typical profiles for the velocity for different values of the radiation parameter. The increasing of the radiation parameter is to increase the dimensionless velocity. This increasing also increase the secondary flow velocity and temperature. This is can be explained by the fact that the effect of radiation is to

Figure 2. Effect of magnetic parameter M on the primary flow velocity V.

Figure 3. Effect of magnetic parameter M on the secondary flow velocity G.

Figure 4. Effect of hall parameter m on the primary flow velocity V.

Figure 5. Effect of the parameter n on the primary flow velocity V.

Figure 6. Effect of the parameter n on the secondary flow velocity G.

Figure 7. Effect of the parameter n on the temperature.

Figure 8. Effect of the parameter N on the temperature.

increase the rate of energy transport to the fluid and accordingly increases the fluid temperature. This increase in the fluid temperature increases the velocity of the fluid particles.

Figures 9-11 show as expected, that the dimensionless velocities and temperature increase as the thermal conductivity parameter increases. This is because as increase the thermal conductivity of the fluid increase. This increase in the fluid thermal conductivity increases the fluid temperature and accordingly the fluid velocity. Figures 12-14 show that the increasing in the temperature ratio parameter tends to increase the dimensionless velocities, and the dimensionless temperature. This result is expected because as increases the temperature difference increases and so the temperature of the fluid Also, it is observed from Figures 15-17 that as the viscosity -temperature parameter increases the dimensionless velocities, increase and the dimensionless temperature decreases. A decrease in the dimensionless temperature means a decrease in the fluid viscosity, which is a gas in this problem. This decrease in the fluid viscosity increase its velocity.

Table 1 shows that the dimensionless wall-velcioty gradient increases as, , , , and increase where as it decreases as increases, the dimensionless wall-velcioty gradient increases as, , , , and increase where as it decreases as increases. Moreover, the dimensionless rate of heat transfer-) increases as, and increase as it decrease as, , , increases.

4. Concluding Remarks

In this paper, we have studied the effects of Hall currents and radiation on the MHD free convective steady lamina boundary layer flow past an isothermal semi-infinite vertical plate, for high temperature differences, the fluid is considered to be electrically conducting in the sence that it is ionized due to radiation.

The fluid density is assumed to vary exponentially and the thermal conductivity linearly with temperature the fluid viscocity is assumed to vary as a reciptocal of a linear function of temperature. Because of the high temperature differences between the fluid and the plate, the Boussinesq approximation is neglected the formula is used to describe the radiative heat flux in the energy equation.

This paper demonstrates the fact that the Boussinesq approximation gives substantial errors in the velocity and temperature distribution for high temperature differences. Therefore, to conclude more accurate results the density variation has to be taken into consideration in the continuity equation, energy equation and all terms of the momentum equation.

Besides, it is observed that:

1) The increasing in the radiation parameter yields to an increasing in the fluid velocities and, the fluid temperature, the dimensionless wall-velocity gradient and the rate of heat transfer from the plate to the fluid.

Figure 9. Effect of the parameter s on the primary flow velocity V.

Figure 10. Effect of the parameter s on the secondary flow velocity G.

Figure 11. Effect of the parameter s on the temperature.

Figure 12. Effect of the parameter θ_w on the primary flow velocity V.

Figure 13. Effect of the parameter θ_w on the secondary flow velocity G.

Figure 14. Effect of the parameter θ_w on the temperature θ.

Figure 15. Effect of the parameter θ_r on the primary flow velocity.

Figure 16. Effect of the parameter θ_r on the secondary flow velocity.

Figure 17. Effect of the parameter θ_r on the temperature θ.

Table 1. Variation of dimensionless wall-velocity gradient and dimensionless rate of heat transfer at the plate with the dimensionless θ_w, N, M, S, m and θ_r for Prandtl number = 0.72 and Gr = 0.5.

2) The increasing in the Hall parameter m yields to a significant increasing in the secondary flow velocity, a slight increasing in the fluid velocities and the fluid temperature the dimensionless wall-velocity gradients and the rate of heat transfer from the plate to the fluid.

3) The increasing in the magnetic parameter yields to an increasing in the fluid temperature, the secondary flow velocity, the dimensionless wall-velocity gradient and the rate of heat transfer from the plate to the fluid and a decreasing in the fluid velocity.

4) The increasing in the thermal conductivity parameter s yields to an increasing in the fluid velocities, the fluid temperature the dimensionless wall-velocity gradient and the plate to the fluid.

5) The increasing in the viscosity-temperature parameter yields to an increasing in the fluid velocities, the dimensionless wall-velocity gradient and a decreasing in the fluid temperature and the rate of the heated transfer from the plate to the fluid.

6) The increasing in the density-temperature parameter yields to an increasing in the fluid velocities and the dimensionless wall-velocity gradient and a decreasing in the fluid temperature and the rate of the heated transfer from the plate to the fluid.

7) The increasing in the temperature ratio parameter yields to an increasing in the fluid velocities and the fluid temperature, the dimensionless wall-velocity gradient and a decreasing in the fluid temperature and the rate of the heated transfer from the plate to the fluid.

Funding

This research is funded by the Deanship of Research and Graduate Studies in Zarqa University/Jordan.

Nomenclature

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Sakiadis, B.C. (1961) Boundary-Layer Behavior on Continuous Solid Surfaces: I. Boundary-Layer Equations for Two-Dimensional and Axisymmetric Flow. AIChE Journal, 7, 26-28. http://dx.doi.org/10.1002/aic.690070108
[2]	Erickson, L.E., Fan, L.T. and Fox, V.G. (1966) Heat and Mass Transfer on Moving Continuous Flat Plate with Suction or Injection. Industrial and Engineering Chemistry Fundamentals, 5, 19. http://dx.doi.org/10.1021/i160017a004
[3]	Cogley, A.C., Vinecti, W.G. and Gilles, S.E. (1968) Differential Approximation for Radiative Transfer in a Nongrey Gas near Equilibrium. AIAA Journal, 6, 551.
[4]	Varjavelu, K. and Hadyinicolaou, A. (1997) Convective Heat Transfer in an Electrically Conducting Fluid at a Stretching Surface with Uniform Free Stream. International Journal of Engineering Science, 35, 1237.
[5]	Griffith, R.M. (1964) Velocity, Temperature, and Concentration Distributions during Fiber Spinning. Industrial and Engineering Chemistry Fundamentals, 3, 245-250. http://dx.doi.org/10.1021/i160011a013
[6]	Chin, D.T. (1975) Mass Transfer to a Continuous Moving Sheet Electrode. Journal of the Electrochemical Society, 122, 643-646. http://dx.doi.org/10.1149/1.2134281
[7]	Gupta, P.S. and Gupta, A.S. (1977) Heat and Mass Transfer on a Stretching Sheet with Suction or Blowing. The Canadian Journal of Chemical Engineering, 55, 744-746. http://dx.doi.org/10.1002/cjce.5450550619
[8]	Gorla, R.S.R. (1978) Unsteady Mass Transfer in the Boundary Layer on a Continuous Moving Sheet Electrode. Journal of the Electrochemical Society, 125, 865-869. http://dx.doi.org/10.1149/1.2131569
[9]	Chakabarti, A. and Gupta, A.S. (1979) Hydromagnetic Flow and Heat Transfer over a Stretching Sheet. The Quarterly of Applied Mathematics, 37, 73.
[10]	Rajagobal, K.R., Na, T.Y. and Gupta, A.S. (1987) A Non-Similar Boundary Layer on a Stretching Sheet in a Non-Newtonian Fluid with Uniform Free Stream. Journal of Mathematical and Physical Sciences, 21, 189.
[11]	Chamkha, A.J. (1999) Hydromagnetic Three-Dimensional Free Convection on a Vertical Stretching Surface with Heat Generation or Absorption. International Journal of Heat and Fluid Flow, 20, 84-92. http://dx.doi.org/10.1016/S0142-727X(98)10032-2
[12]	Elbashbashy, E.M.A. (1998) Heat Transfer over a Stretching Surface with Variable Surface Heat Flux. Journal of Physics D: Applied Physics, 31, 1951.
[13]	Lai, F.C. and Kulaki, F.A. (1990) The Effect of Variable Viscosity on Convective Heat Transfer along a Vertical Surface in a Saturated Porous Medium. International Journal of Heat and Mass Transfer, 33, 1028-1031. http://dx.doi.org/10.1016/0017-9310(90)90084-8
[14]	Jaber, K.K. and Al-Athari, F. (2014) Hall Current Effect on Unsteady MHD Flow between Two Horizontal Plates with Constant Suction. IJARET, 5, 1.
[15]	Pop, I. and Watanabe, T. (1993) Magnetohydrodynamic Free Convection Flow over a Wedge in the Presence of a Transverse Magnetic Field. International Communications in Heat and Mass Transfer, 20, 871-881. http://dx.doi.org/10.1016/0735-1933(93)90040-3
[16]	Abo-Eldahab, E.M. (2001) Hall Effects on Magnetohydrodynamic Free-Convection Flow at a Stretching Surface with a Uniform Free-Stream. Physica Scripta, 63, 29-35. http://dx.doi.org/10.1238/Physica.Regular.063a00029
[17]	Khaled, K. (2013) Non Newtonian Fluid Flow under the Effect of Chemical Reaction and Ion and Hall Currents over a Moving Cylinder. Jaber Journal of Purity, Utility Reaction and Environment, 2, 1-13.
[18]	El-Hakiem, M.A., Mohammadein, A.A. and El-Kabeir, S.M.M. (1999) Joule Heating Effects on Magnetohydrodynamic Free Convection Flow of a Micropolar Fluid. International Communications in Heat and Mass Transfer, 26, 219-227.
[19]	Sparrow, E.M. and Cess, R.D. (1978) Radiation Heat Transfer. Hemisphere Publishing Corporation, Washington DC.
[20]	Kakac, S. and Yener, Y. (1995) Convective Heat Transfer. CRC Press, Boca Raton, 312.
[21]	Aboeldahab, E.M. (2004) The Effects of Temperature-Dependent Fluid Properties on Free Convective Flow along a Semi-Infinite Vertical Plate by the Presence of Radiation. Heat Mass Transfer, 41, 163.
[22]	Jaber, K.K. (2012) Transient MHD Mixed Double Diffusive Convection along a Vertical Plate Embedded in a Non-Darcy Porous Medium with Suction or Injection. Journal of Mathematics and Statistics, 8, 1.
[23]	Aboeldahab, E.M. and El-Gendy, M.S. (2000) Radiation Effect on Convective Heat Transfer in an Electrically Conducting Fluid at a Stretching Surface with Variable Viscosity and Uniform Free Stream. Physica Scripta, 62, 321. http://dx.doi.org/10.1238/Physica.Regular.062a00321
[24]	Aboeldahab, E.M. and Salem, A.M. (2001) Radiation Effect on MHD Free Convection Flow of a Gas Past a Semi-Infinite Vertical Plate with Variable Viscosity. International Journal of Computational Fluid Dynamics, 14, 243-252. http://dx.doi.org/10.1080/10618560108940727