1. Introduction
It is well known that many mathematical physics problems can be put into the perspective of infinite dimensional systems, which can be equivalently described by 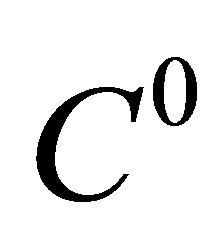 semigroups in proper function spaces. One important object to describe the long time dynamics of an infinite dimensional system is the global attractor, which is a connected and compact invariant set in some function space, and which attracts all bounded sets.
semigroups in proper function spaces. One important object to describe the long time dynamics of an infinite dimensional system is the global attractor, which is a connected and compact invariant set in some function space, and which attracts all bounded sets.
To show the existence of the global attractor, one normally needs to verify:
1) there exists an absorbing set, and
2) the semigroup is uniformly compact.
However, it is difficult or even impossible to verify the uniform compactness of the semigroup for many problems. In [1], the authors use the measure of noncompactness of a set to introduce a new concept of compactness called  -limit compact, then they show that there exists a global attractor for a
-limit compact, then they show that there exists a global attractor for a  semigroup if and only if:
semigroup if and only if:
1) there is an absorbing set, and
2) the semigroup is  -limit compact.
-limit compact.
A well-known result (see [2-6]) is that a continuous semigroup has a global attractor if and only if:
1) it has a bounded absorbing set, and
2) it is asymptotically compact.
Furthermore, in [7], the author introduce the concept of asymptotically null and show that a lattice system has a global attractor if and only if:
1) it has a bounded absorbing set, and
2) it is asymptotically null.
Our main motivation of this paper is to prove that asymptotically compact Û  -limit compact, and then we prove that the conditions in [1,7] are equivalent directly in
-limit compact, and then we prove that the conditions in [1,7] are equivalent directly in .
.
The concept of pullback random attractors for random dynamical systems, which is an extension of the attractors theory of deterministic systems, was introduced by the authors in [8-10]. We point out that our work in this paper also can be extended to pullback attractors.
2. Measure of Noncompactness and Its Properties
In this section, we recall the concept of measure of noncompactness and recapitulate its basic properties; see [11].
Definition 2.1 Let M be a metric space and A be a bounded subset of M. The measure of noncompactness 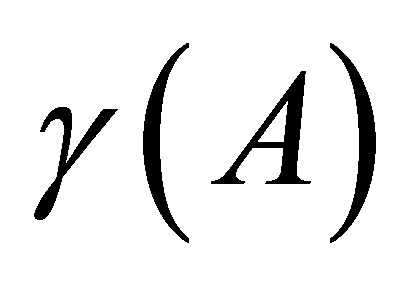 of A is defined by
of A is defined by
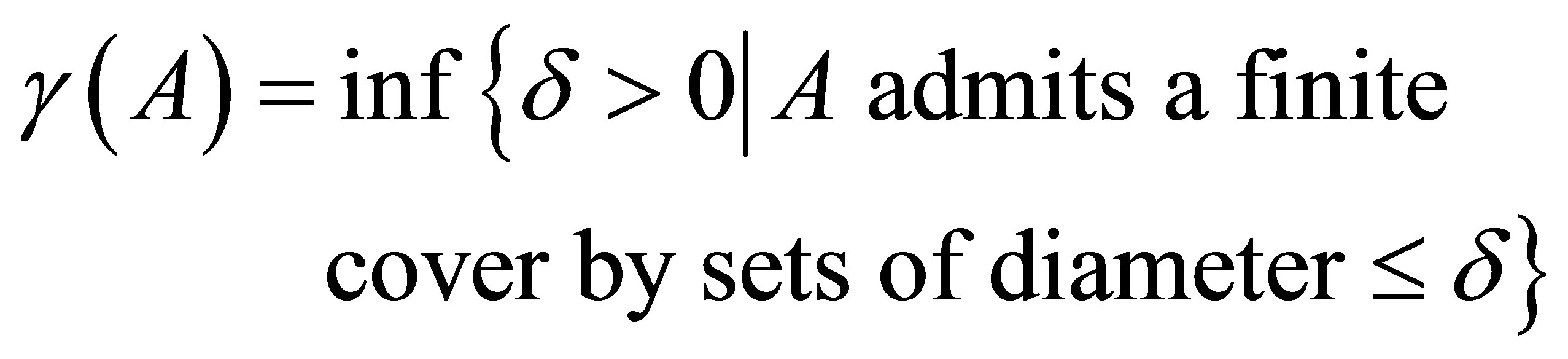 .
.
Lemma 2.1 Let M be a complete metric space, and 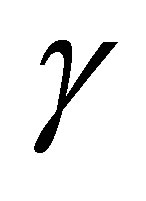 be the measure of noncompactness of a set.
be the measure of noncompactness of a set.
1) 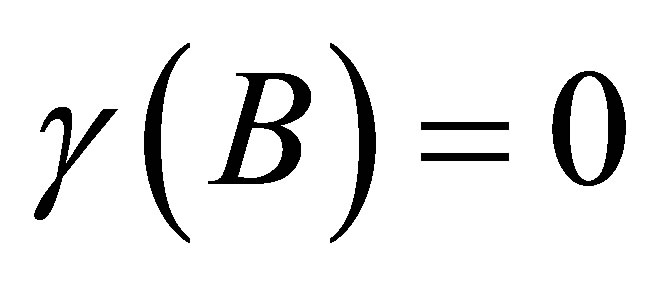 if and only if
if and only if 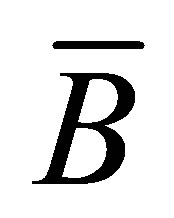 is compact;
is compact;
2) If M is a Banach space, then ;
;
3)  whenever
whenever ;
;
4) ;
;
5) .
.
Proof. 1) (a) If  is compact, then
is compact, then  is precompact.
is precompact. 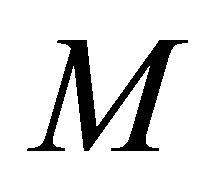 is a complete metric space, thus for any
is a complete metric space, thus for any , there exists a finite subset
, there exists a finite subset 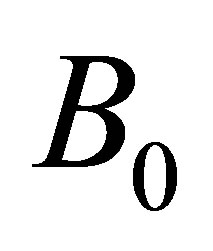 of
of 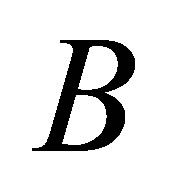 such that the balls of radii
such that the balls of radii 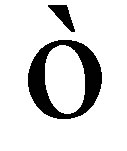 centered at
centered at  form a finite covering of
form a finite covering of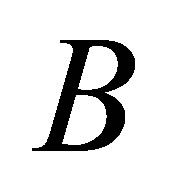 . By Definition 2.1,
. By Definition 2.1,  admits a finite cover by sets of diameter
admits a finite cover by sets of diameter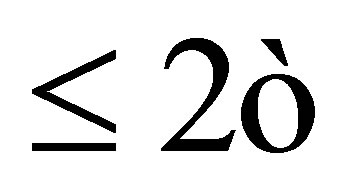 . The arbitrariness of
. The arbitrariness of  implies that
implies that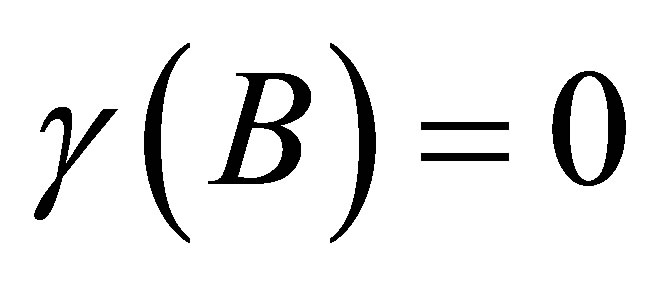 .
.
(b) On the other hand, if , then by Definition 2.1, we have that for any
, then by Definition 2.1, we have that for any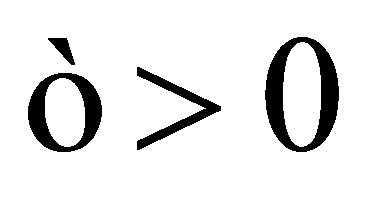 ,
,  admits a finite cover by sets of diameter
admits a finite cover by sets of diameter . So for any
. So for any ,
,  always has a finite
always has a finite 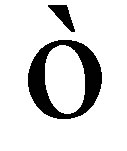 -net. Then
-net. Then  is totally bounded.
is totally bounded.  is complete, thus
is complete, thus 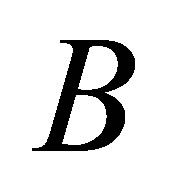 is precompact, and
is precompact, and  is compact.
is compact.
2) If 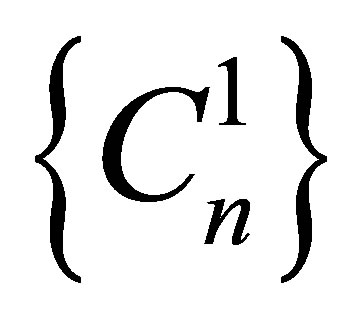 is a finite cover of
is a finite cover of , and
, and 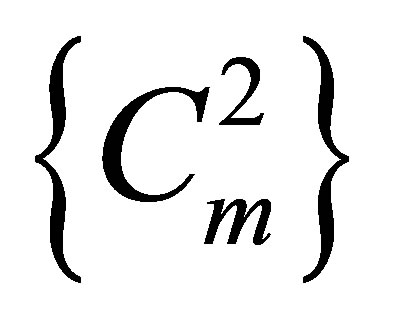 is a finite cover of
is a finite cover of , then
, then 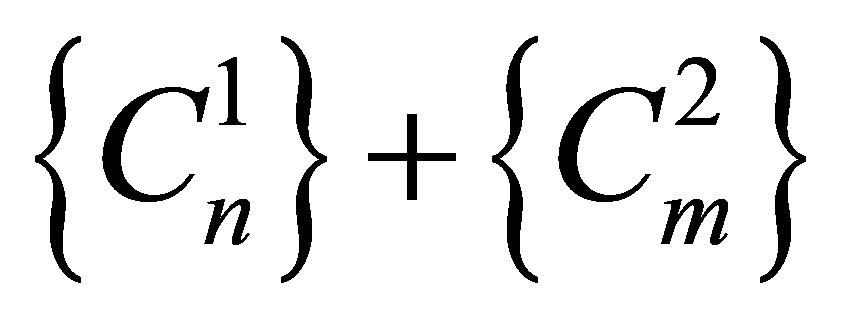 is a finite cover of
is a finite cover of , thus
, thus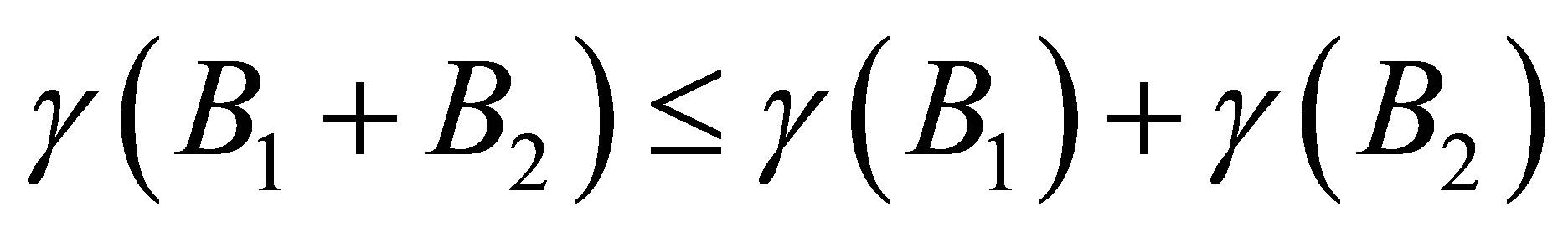 .
.
3) If , then the finite cover of
, then the finite cover of  must be a finite cover of
must be a finite cover of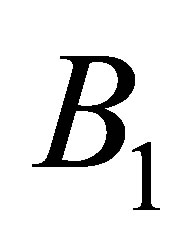 , so
, so .
.
4) (a) The finite cover of  must be a finite cover of both of
must be a finite cover of both of 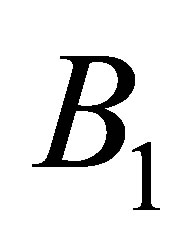 and
and . So we have
. So we have  and
and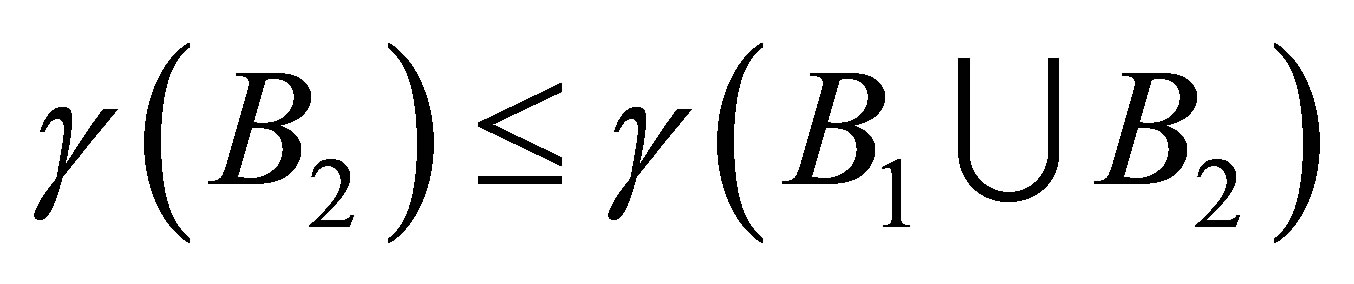 . Thus
. Thus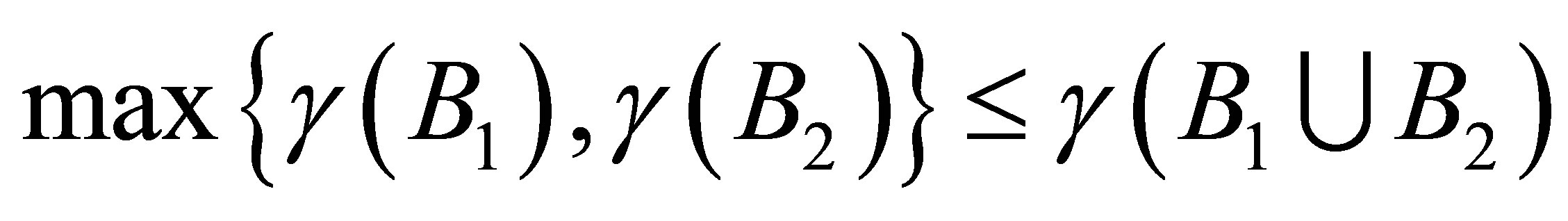 .
.
(b) For any , we can find finite covers
, we can find finite covers  of
of  and
and  of
of 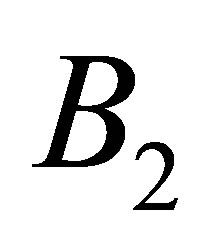 with the diameter of
with the diameter of  and
and 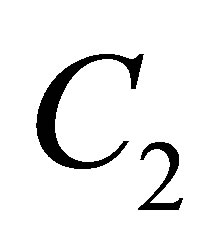 less than
less than . But
. But 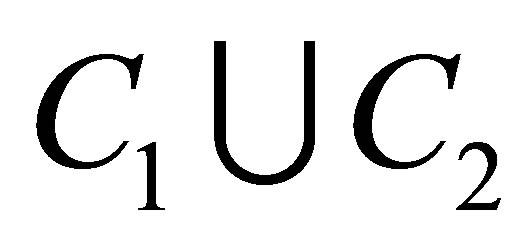 is a cover of
is a cover of 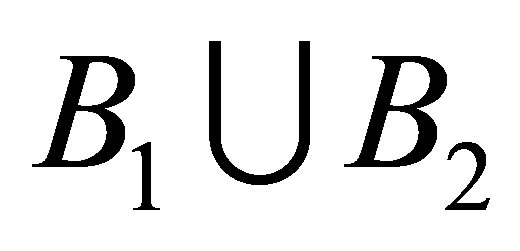 and the diameter of
and the diameter of 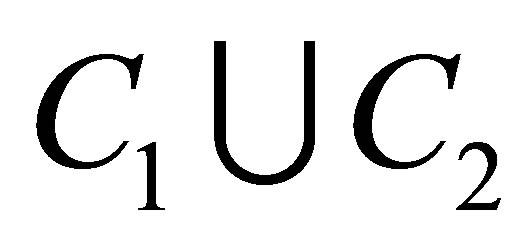 is less than
is less than . Hence
. Hence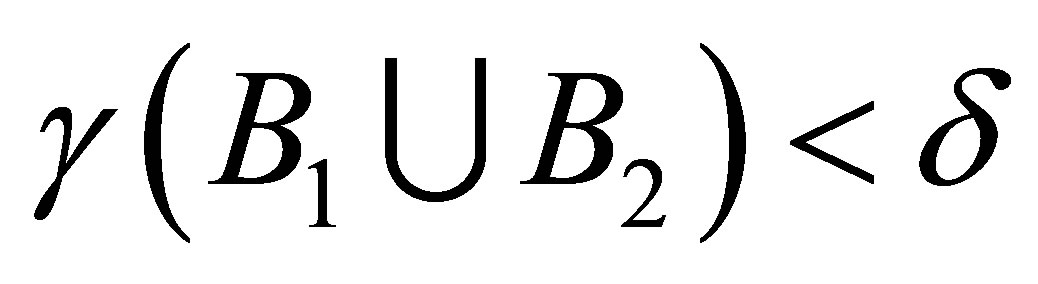 . So
. So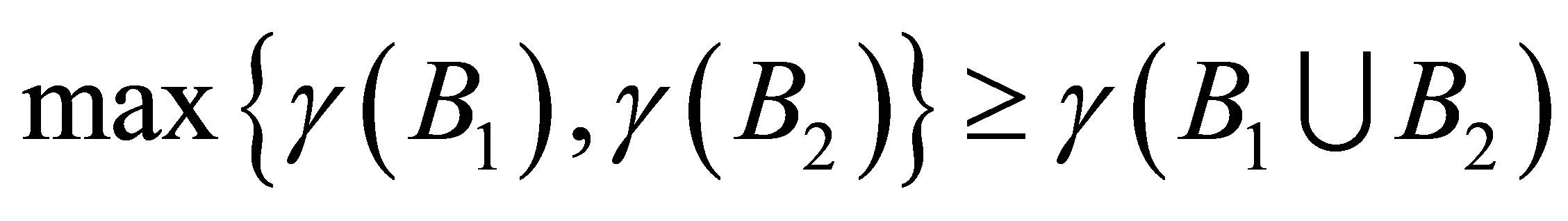 .
.
5) Since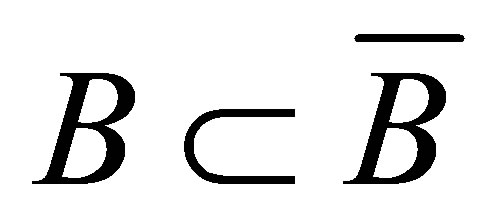 , then
, then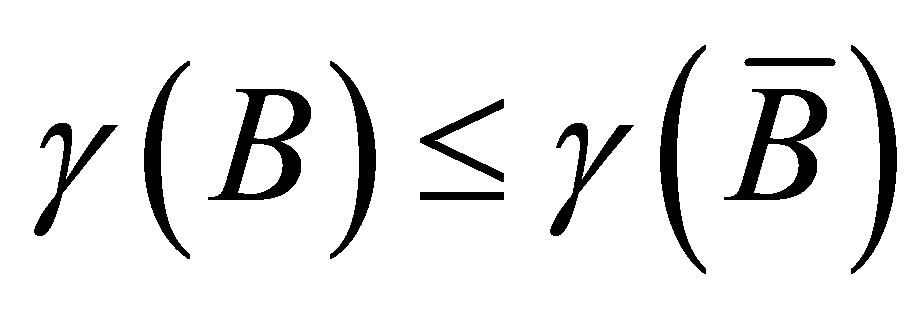 . For any
. For any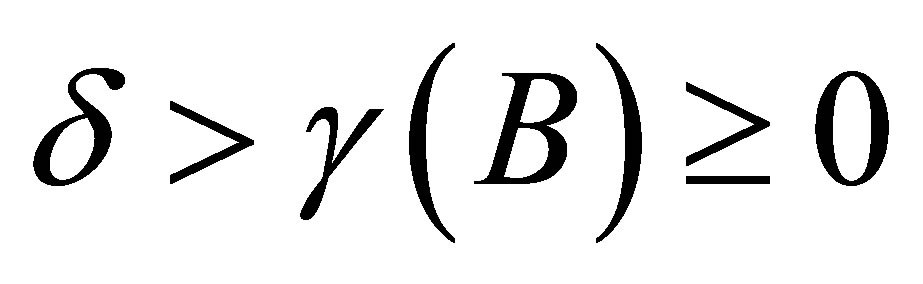 ,
,  has a finite cover by sets of diameter
has a finite cover by sets of diameter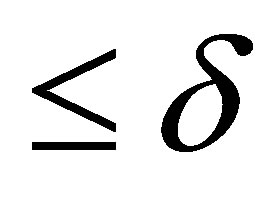 . For any
. For any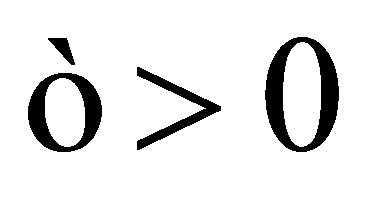 ,
, 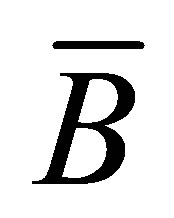 has a finite cover by sets of diameter
has a finite cover by sets of diameter . From the arbitrariness of
. From the arbitrariness of 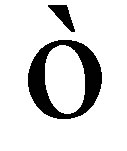 and Definition 2.1, we have
and Definition 2.1, we have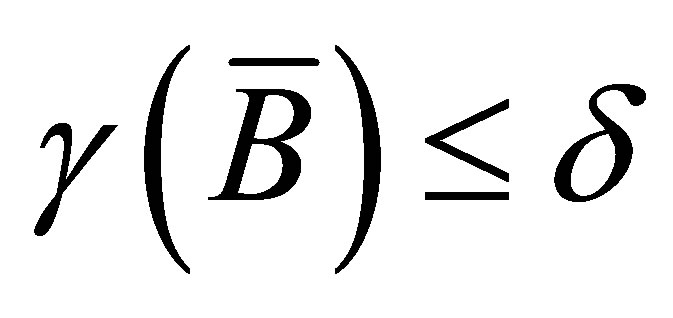 . Thus
. Thus . So
. So .
.
3. Main Results
In this section, firstly we recall some basic definitions in [1,7], then we show that the two necessary and sufficient conditions for the existence of global attractors for semigroups are equivalent directly.
Definition 3.1 Let 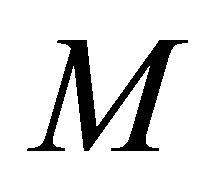 be a complete metric space. A one parameter family
be a complete metric space. A one parameter family  of maps
of maps
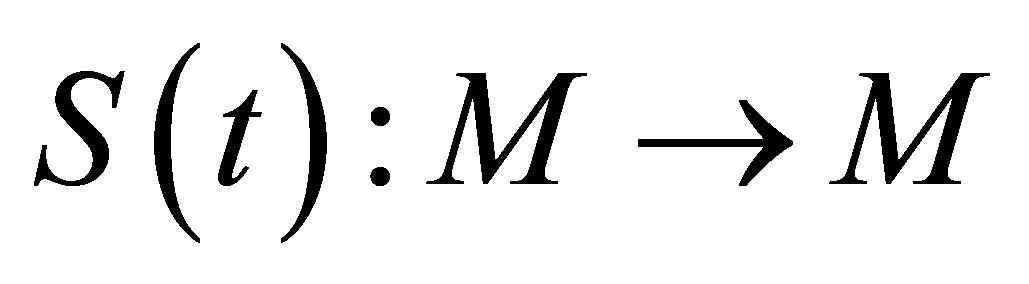 ,
, 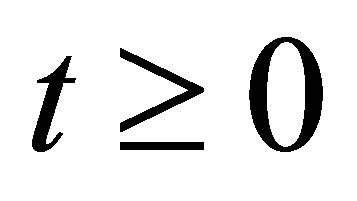 is called a
is called a 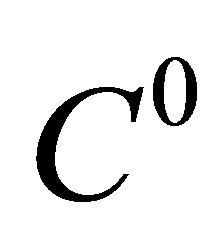 semigroup if 1)
semigroup if 1)  is the identity map on M2)
is the identity map on M2)  for all
for all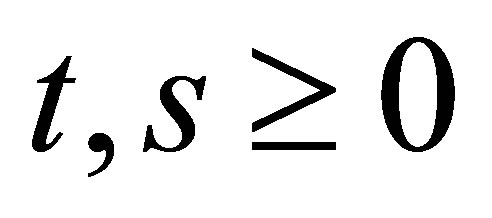 3) the function
3) the function  is continuous at each point
is continuous at each point .
.
Definition 3.2 Let 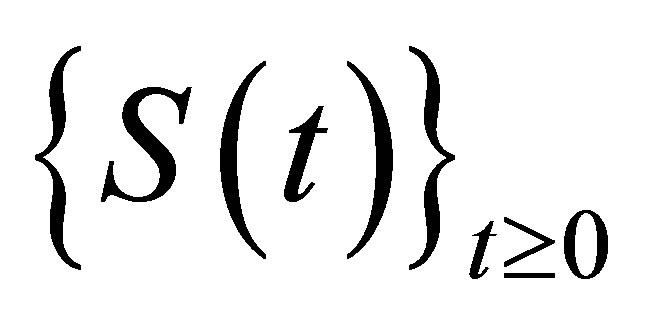 be a
be a  semigroup in a complete metric space
semigroup in a complete metric space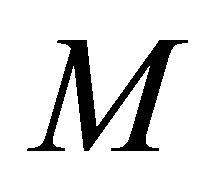 . A subset
. A subset 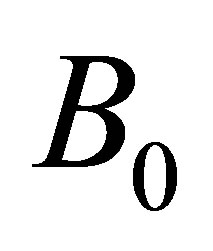 of
of  is called an absorbing set in
is called an absorbing set in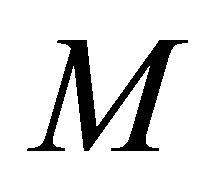 , if for any bounded subset
, if for any bounded subset 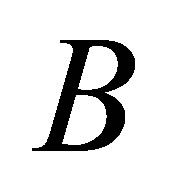 of
of , there exists some
, there exists some  such that
such that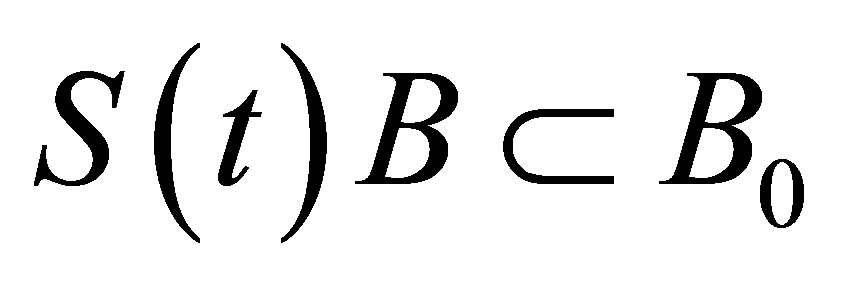 , for all
, for all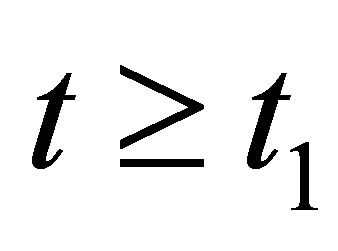 .
.
Definition 3.3 A  semigroup
semigroup  in a comple te metric space
in a comple te metric space  is called
is called  -limit compact, if for every bounded subset
-limit compact, if for every bounded subset 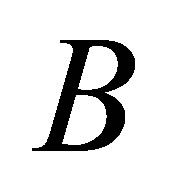 of
of  and any
and any , there exists
, there exists  such that
such that
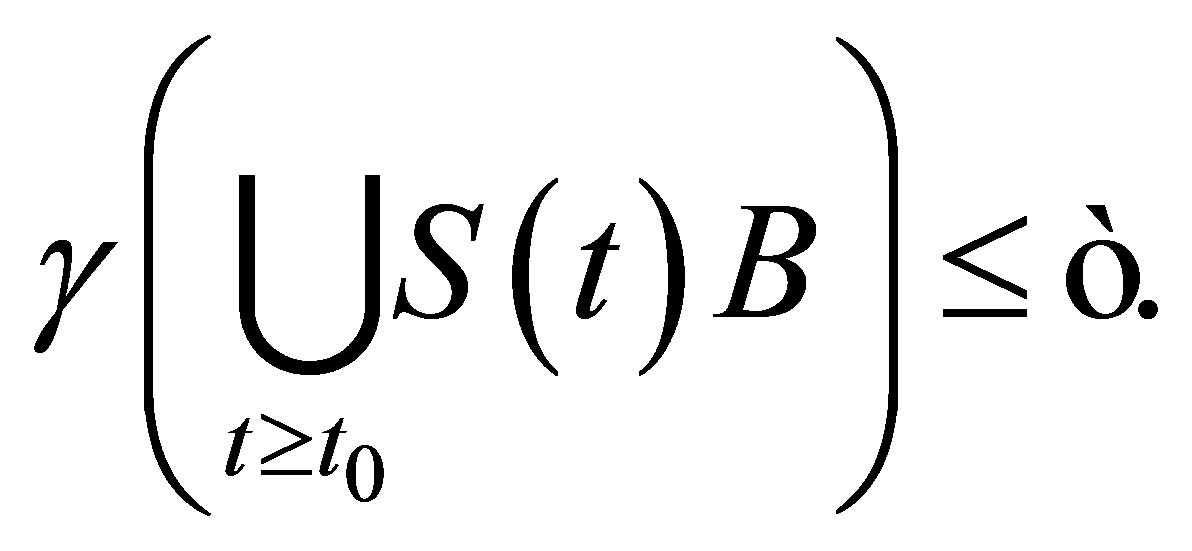
Definition 3.4 A  semigroup
semigroup  in a complete metric space
in a complete metric space  is called asymptotically compact if, for every bounded subset
is called asymptotically compact if, for every bounded subset , for any
, for any  and any
and any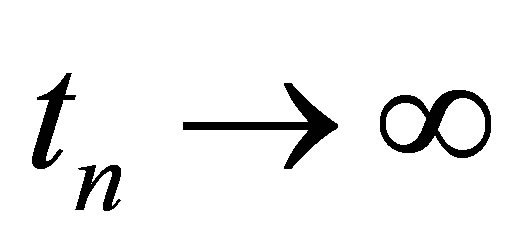 ,
, 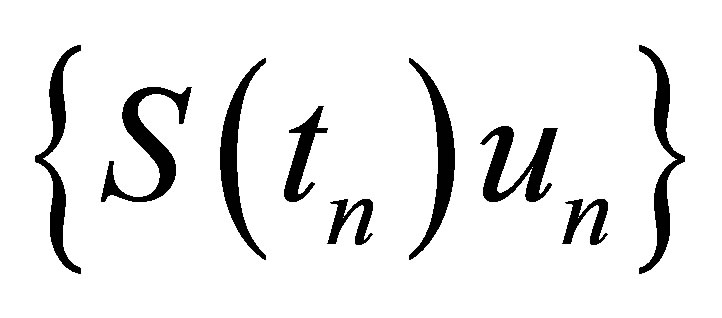 has a convergent subsequence.
has a convergent subsequence.
Let  be a positive smooth function on R and
be a positive smooth function on R and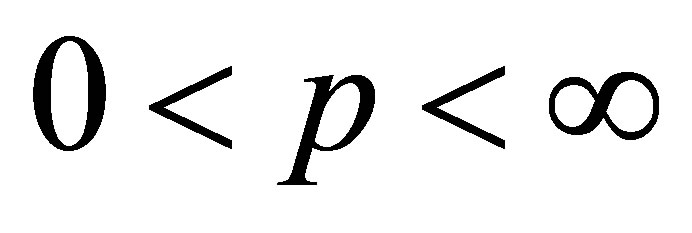 . Then define a weighted
. Then define a weighted 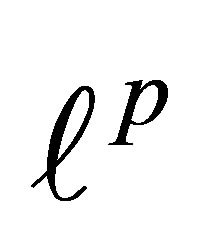 space as
space as
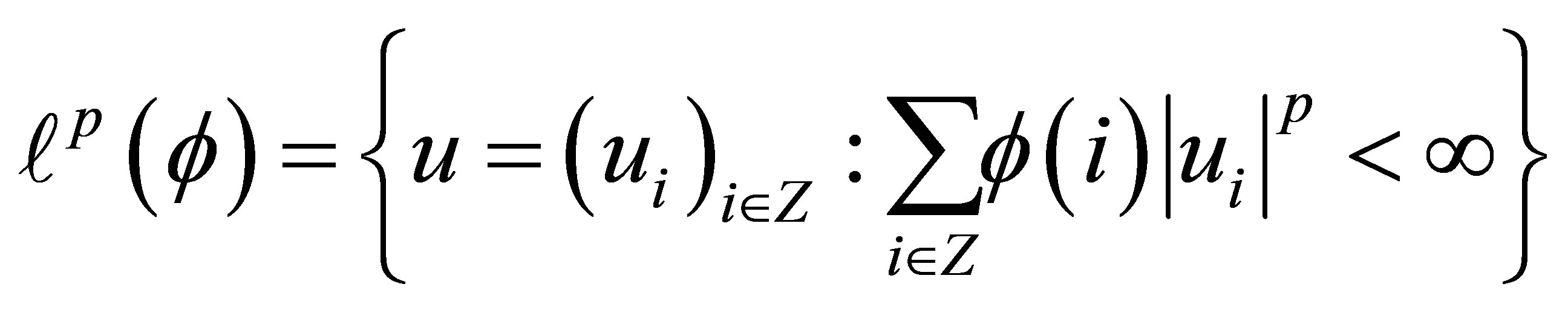
with norm .
.
Definition 3.5 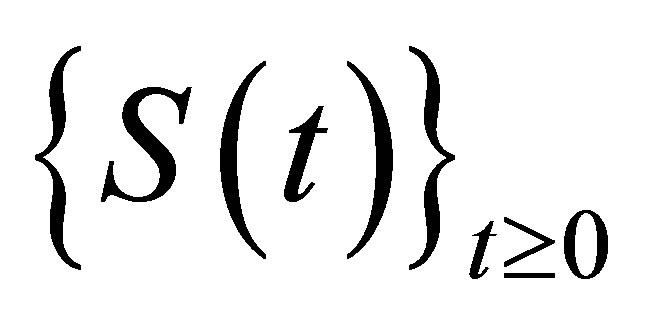 is said to be asymptotically null in
is said to be asymptotically null in  if for any
if for any  bounded in
bounded in  and
and , the following holds
, the following holds

Theorem 3.1 Let 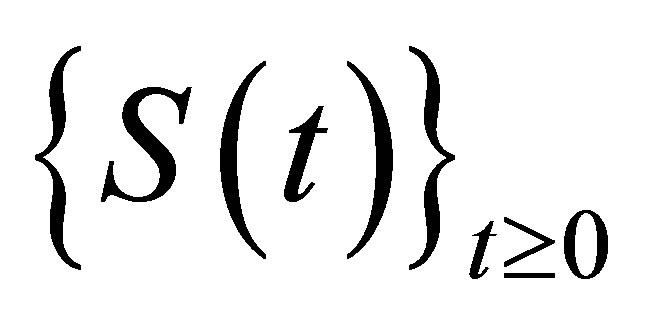 be a
be a  semigroup in a complete metric space M, then we can have:
semigroup in a complete metric space M, then we can have:
 is
is  -limit compact Û
-limit compact Û 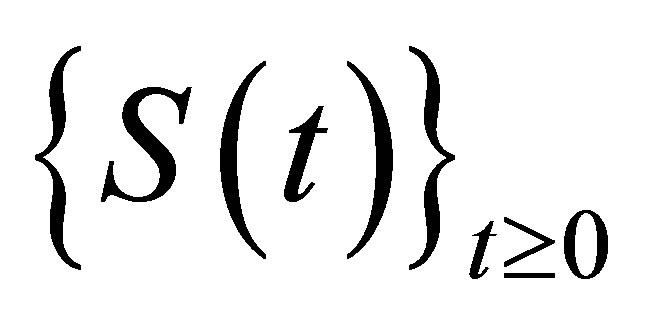 is asymptotically compact.
is asymptotically compact.
Proof. First, we prove the necessity.
It suffices to prove that for every bounded subset , for any
, for any , there exists
, there exists , such that
, such that

Assume otherwise, then there exists a bounded subset  and
and , such that for every
, such that for every  we have
we have

We take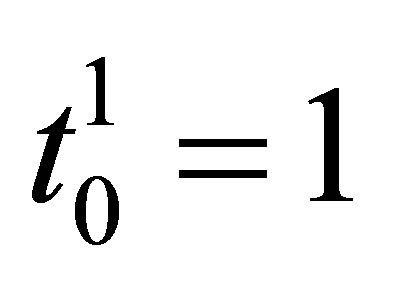 , then
, then . Let
. Let  and take
and take .
.
Let  then
then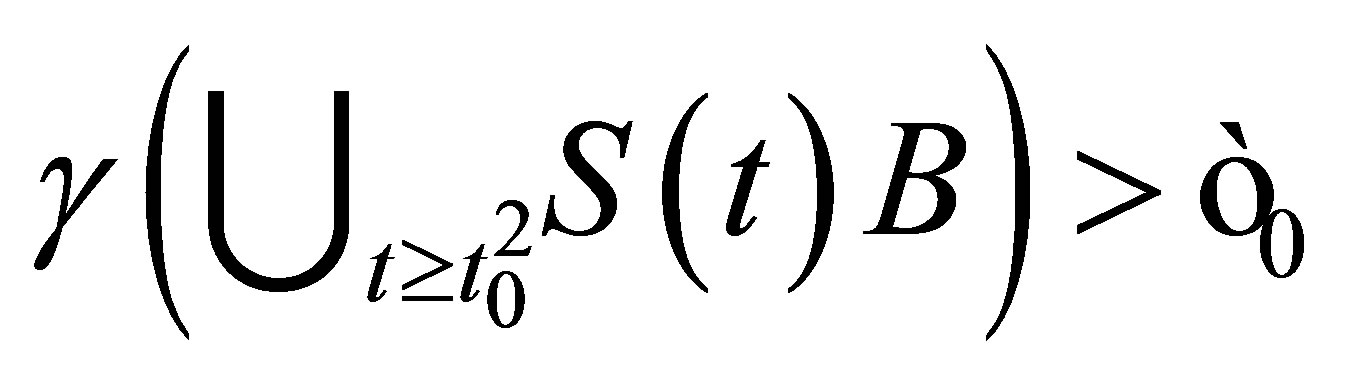 . By the definition of measure of noncompactness,
. By the definition of measure of noncompactness,
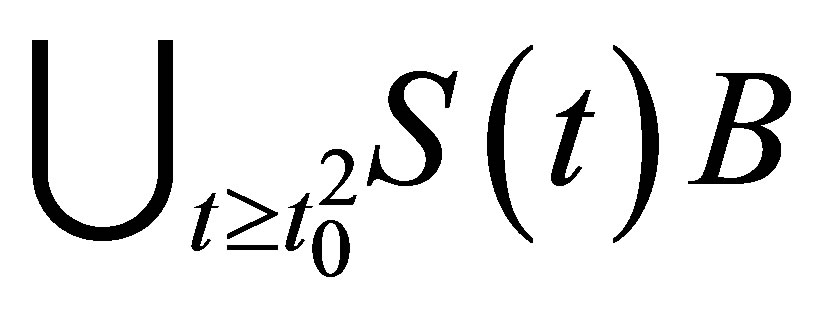 has no finite covering of balls of radii
has no finite covering of balls of radii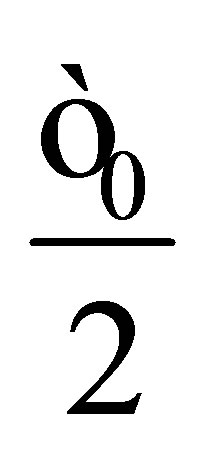 .
.
Thus there exists  and
and  such that
such that

Otherwise  is the finite
is the finite 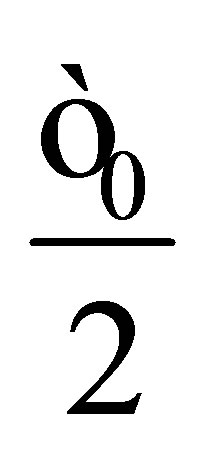 -net of
-net of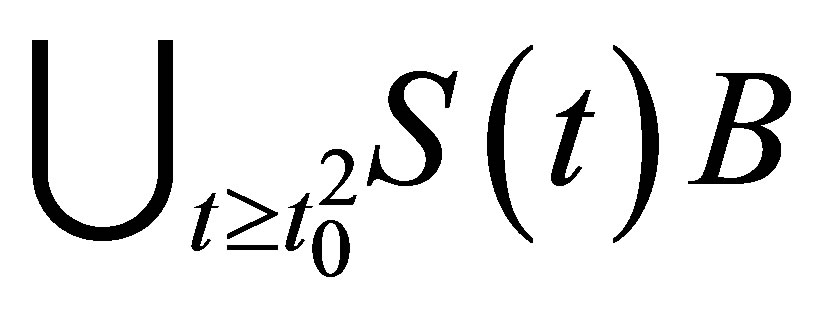 .
.
Next we take 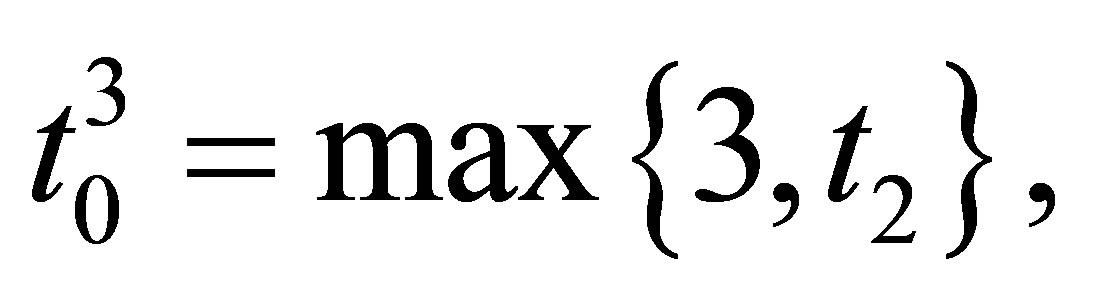 hence
hence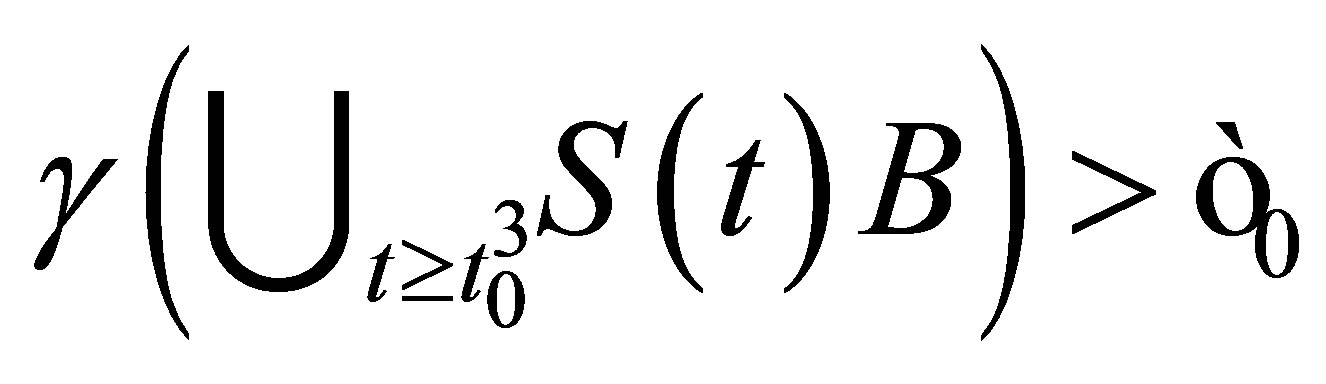 . That is to say
. That is to say 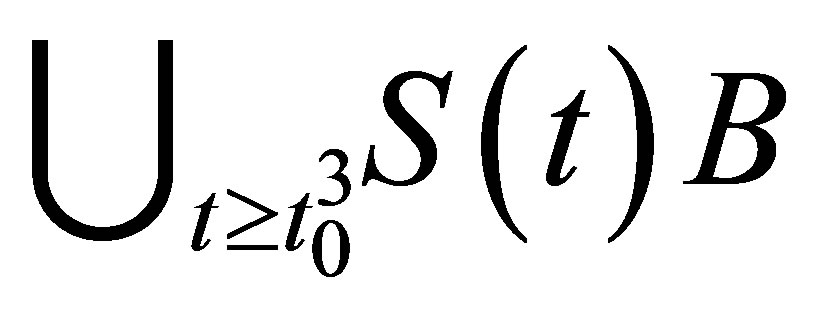 has no finite
has no finite 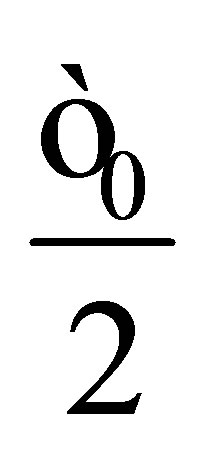 -net. Thus there exists
-net. Thus there exists  and
and  such that
such that
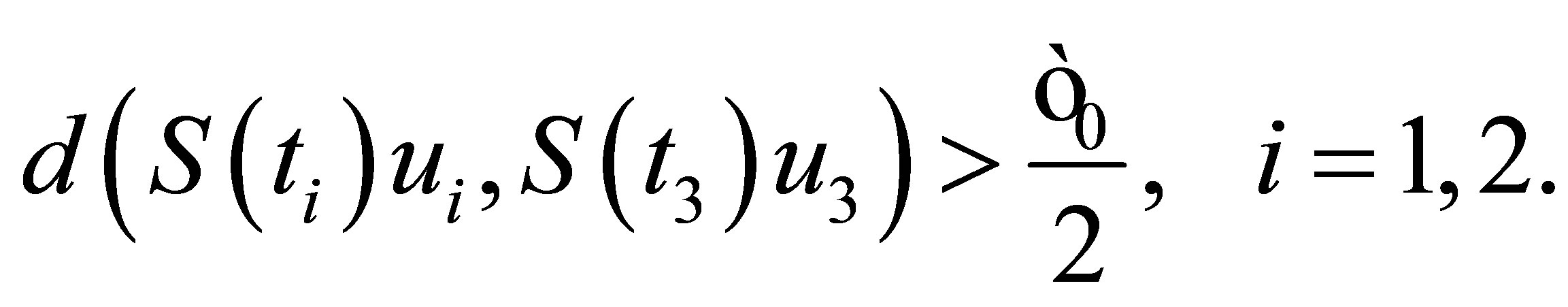
Otherwise 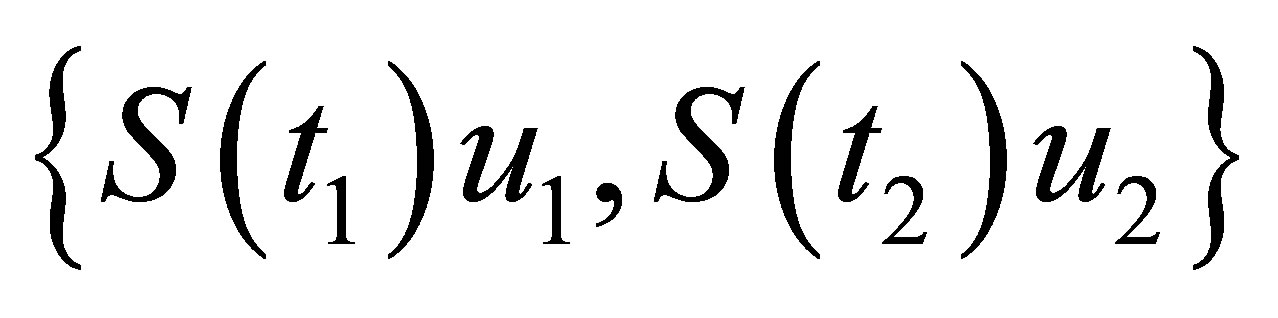 is the finite
is the finite  -net of
-net of .
.
Repeat the previous procedure, then we have the sequence  which satisfies
which satisfies
 (1)
(1)
By the way of taking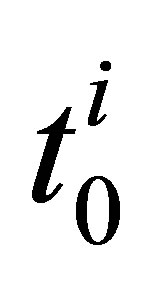 , and
, and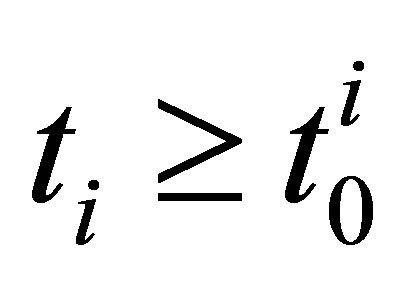 , we have
, we have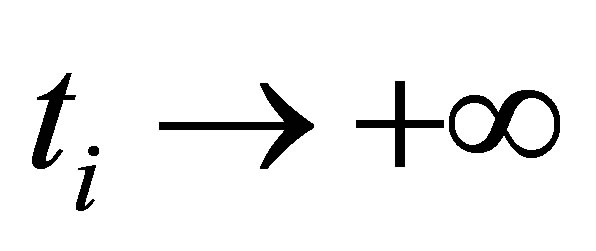 . Since
. Since 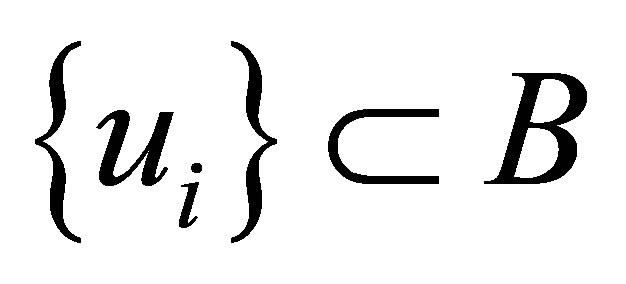 and
and 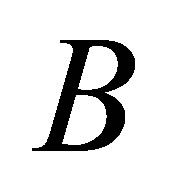 is a bounded subset of
is a bounded subset of ,
, 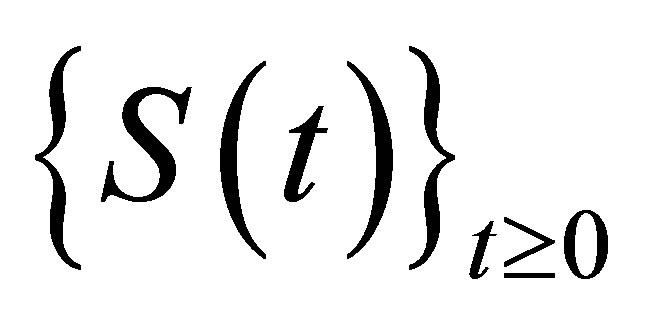 is asymptotically compact. Therefore
is asymptotically compact. Therefore  has a convergent subsequence. This gives contradiction to (1).
has a convergent subsequence. This gives contradiction to (1).
Thus  is
is  -limit compact.
-limit compact.
Next, we prove the sufficiency.
We need to prove that for every bounded subset , for any
, for any 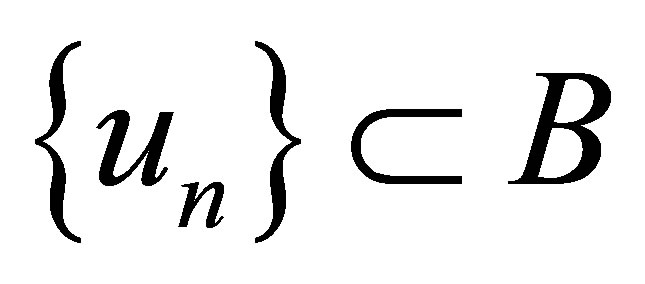 and any
and any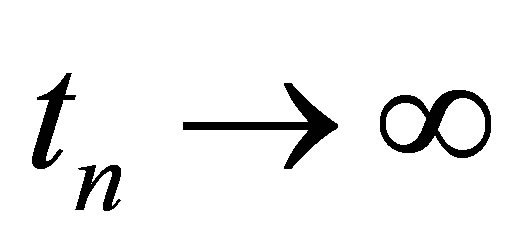 ,
, 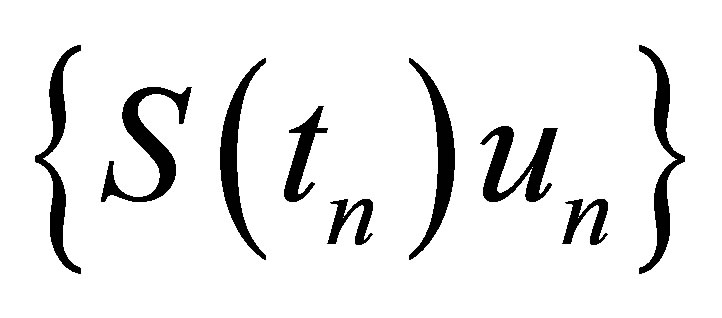 has a convergent subsequence.
has a convergent subsequence.
Since 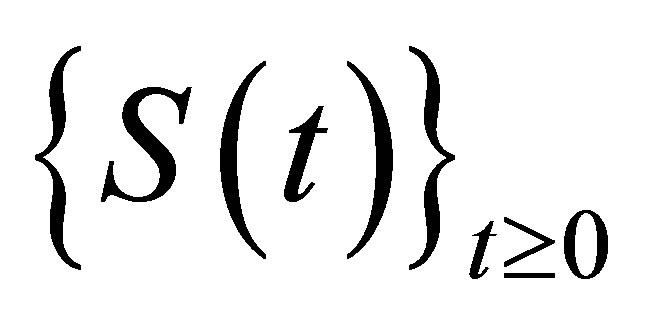 is
is  -limit compact, then for the bounded subset
-limit compact, then for the bounded subset  above, for any
above, for any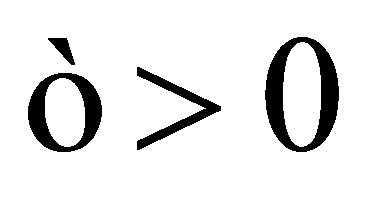 , there exists
, there exists 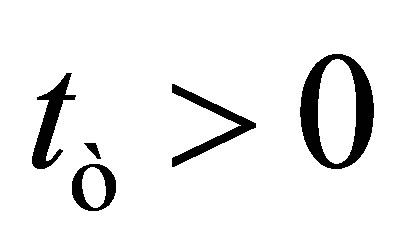 such that
such that

For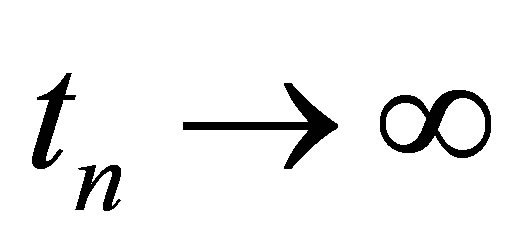 , there exists
, there exists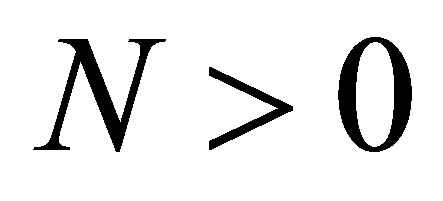 , such that
, such that 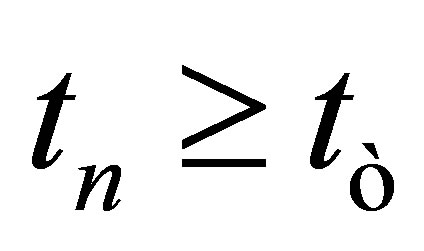 when
when .
.  implies
implies
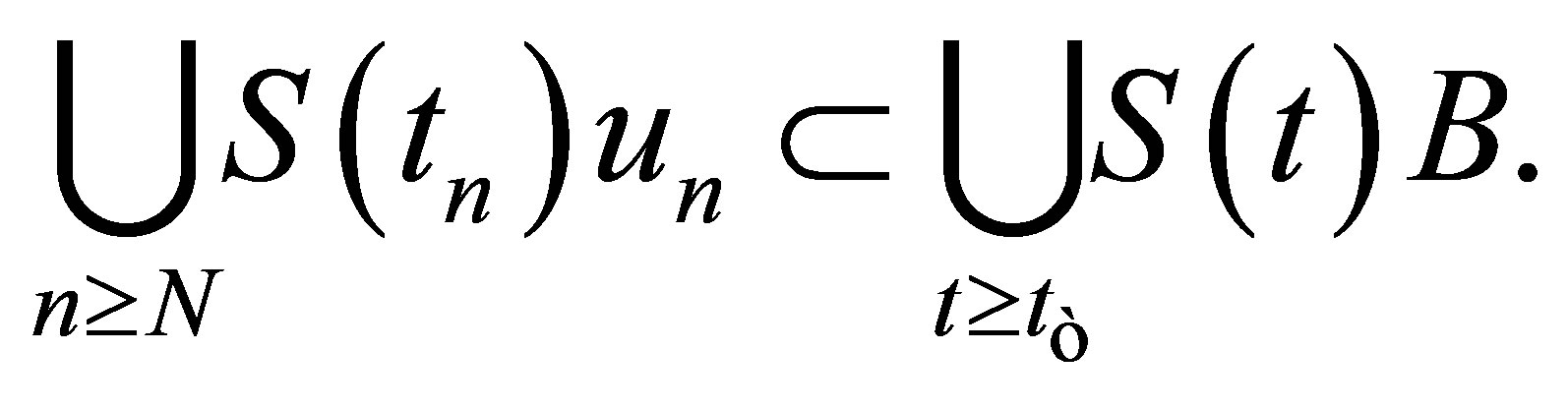
Property (3) of the measure of noncompactness in Lemma 2.1 shows that
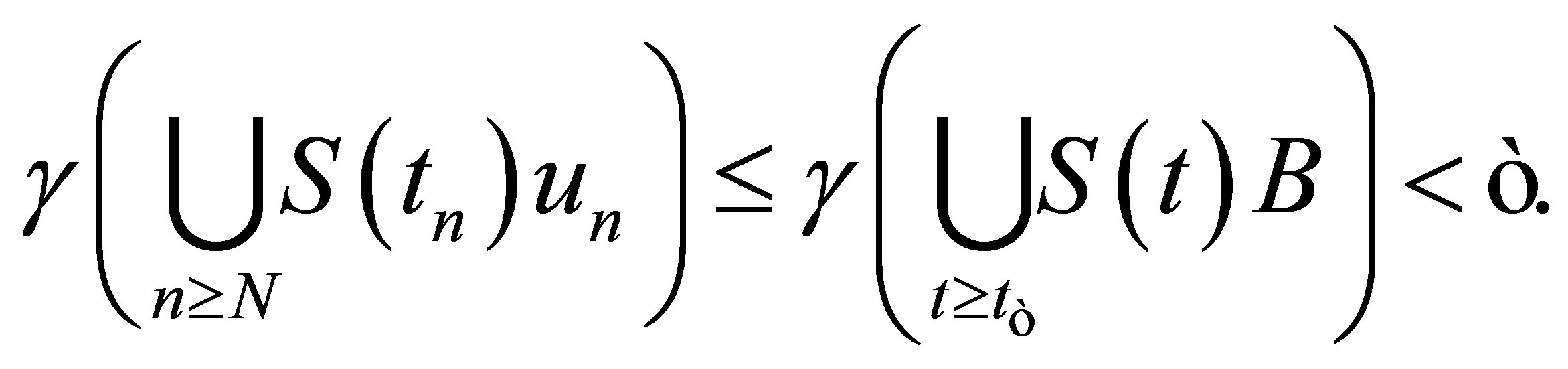
So . Notice that
. Notice that 
contains only a finite number of elements (where  is fixed such that
is fixed such that  as
as ).
).
Using properties in Lemma 2.1, we have

Thus

From the arbitrariness of , it has
, it has
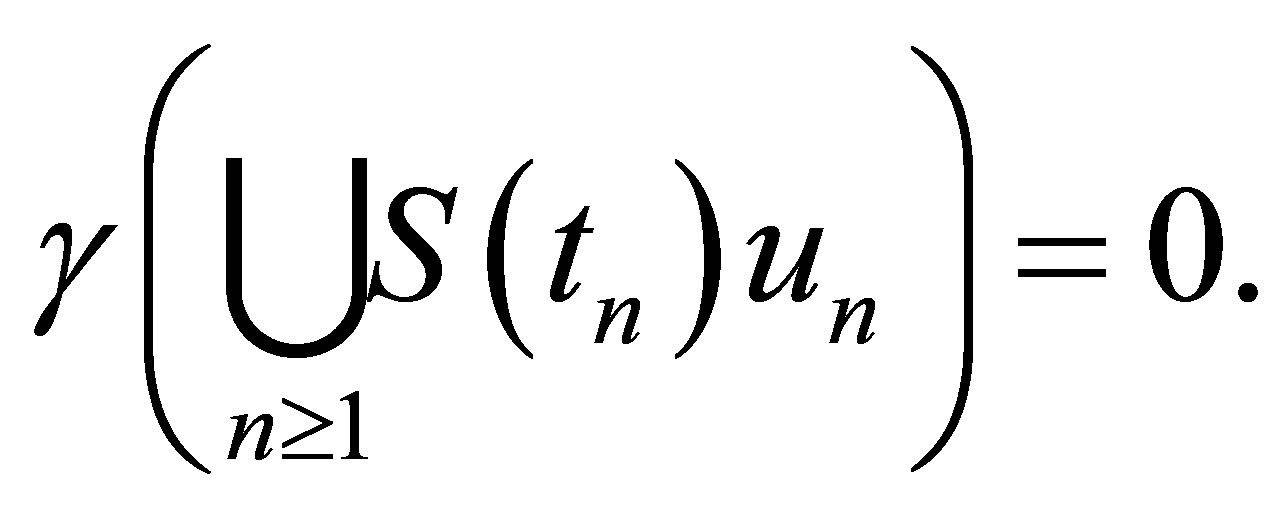
Hence  is precompact. Thus
is precompact. Thus 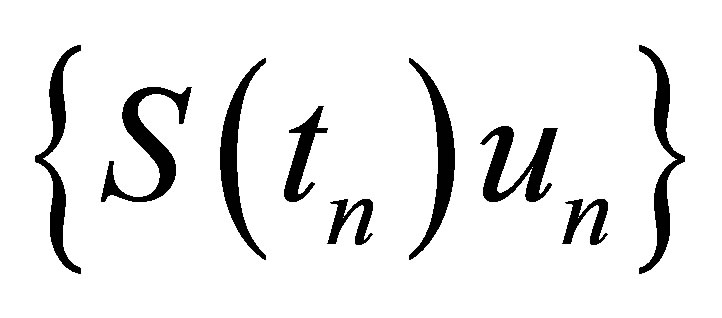 has a convergent subsequence. Therefore
has a convergent subsequence. Therefore  is asymptotically compact. This completes the proof of Theorem.
is asymptotically compact. This completes the proof of Theorem.
Corollary 1 Let 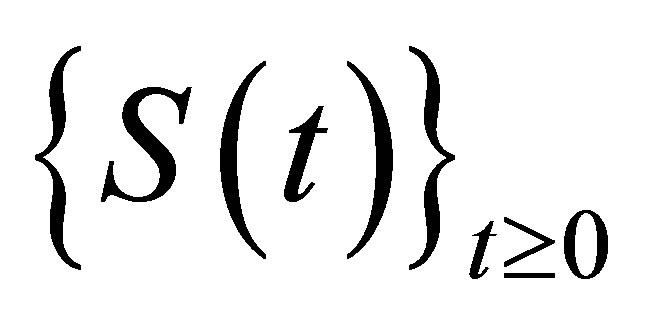 be a semigroup of continuous operators in
be a semigroup of continuous operators in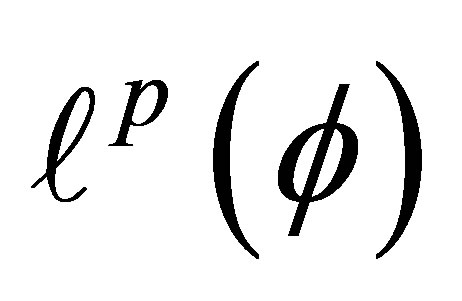 . Then
. Then  has a bounded absorbing set and it is asymptotically null in
has a bounded absorbing set and it is asymptotically null in  has a bounded absorbing set and it is
has a bounded absorbing set and it is  -limit compact.
-limit compact.
Proof. By Corollary 3.4 in [7], we have 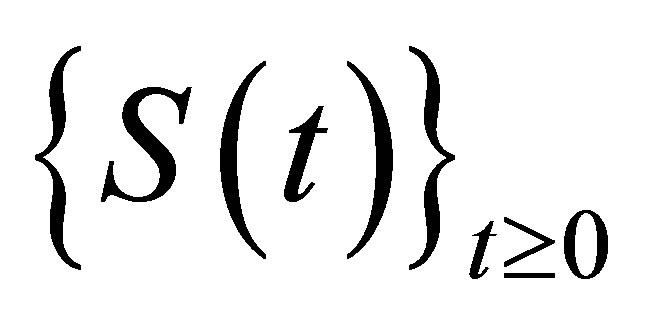 is asymptotically compact in
is asymptotically compact in  if and only if
if and only if  is asymptotically null in
is asymptotically null in 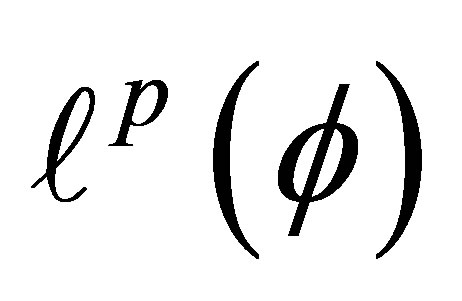 and
and 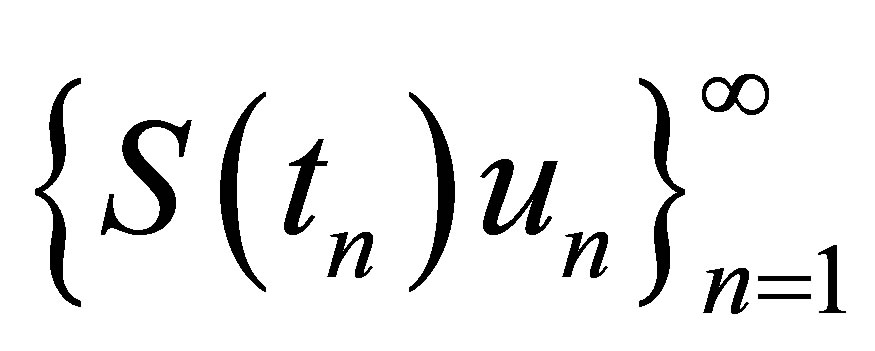 is bounded in
is bounded in 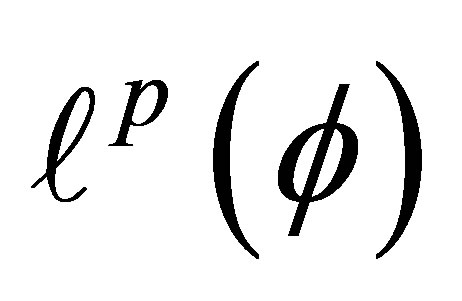 provided
provided 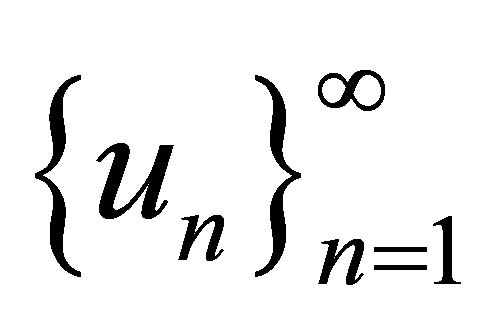 is bounded and
is bounded and .
.
Using the Theorem 3.1 above , we have 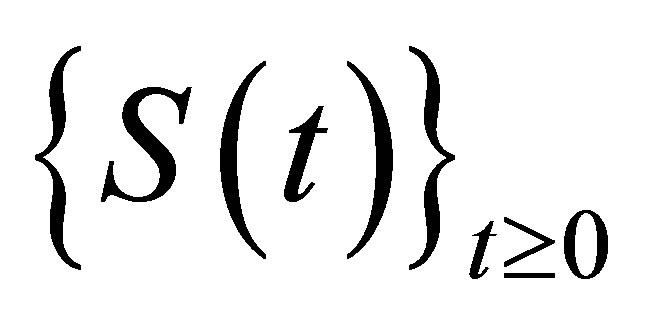 is
is  -limit compact in
-limit compact in  if and only if
if and only if 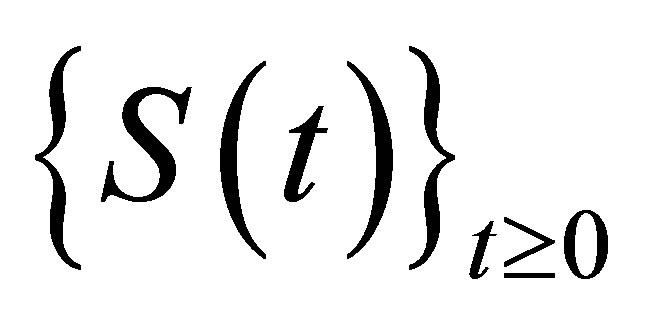 is asymptotically null in
is asymptotically null in 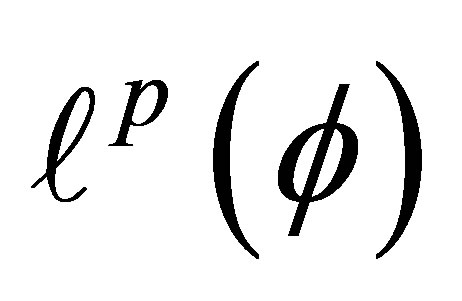 and
and  is bounded in
is bounded in 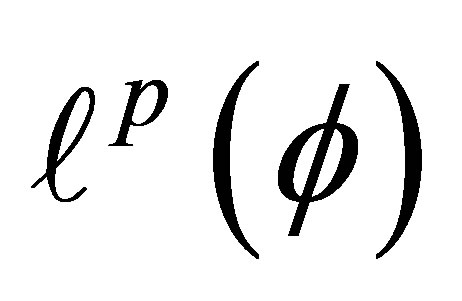 provided
provided  is bounded and
is bounded and . Thus the necessity of the corollary is obvious.
. Thus the necessity of the corollary is obvious.
If 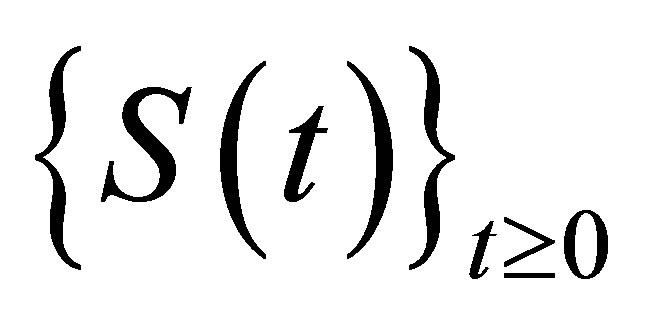 has a bounded absorbing set,
has a bounded absorbing set, 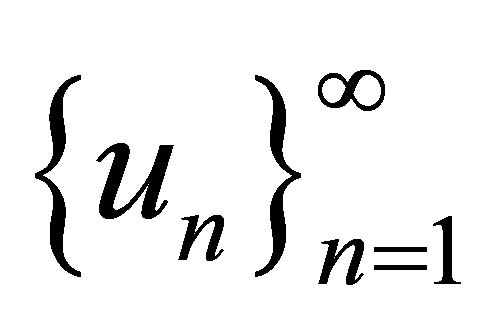 is bounded and
is bounded and , then there exists
, then there exists 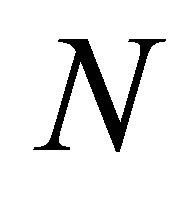 such that
such that 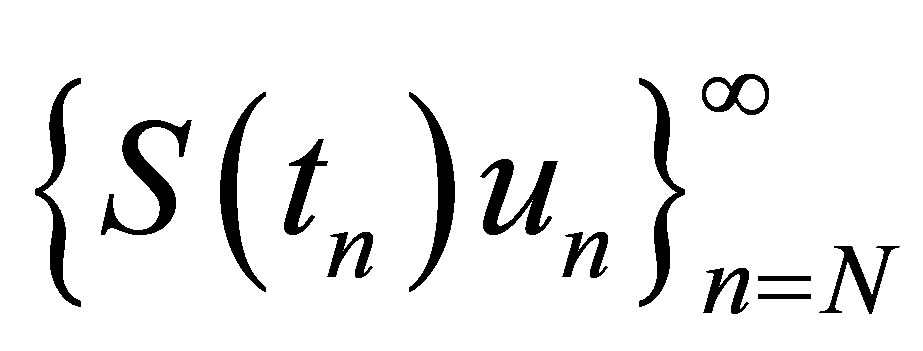 is contained in the bounded absorbing set.
is contained in the bounded absorbing set. 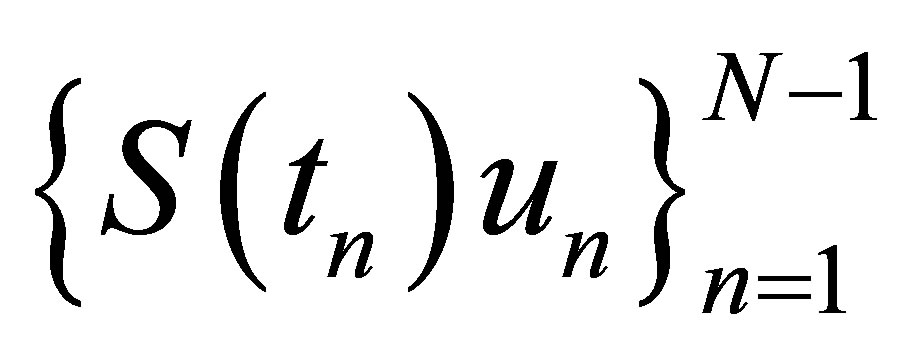 is a finite set in
is a finite set in , so it is bounded. Thus
, so it is bounded. Thus 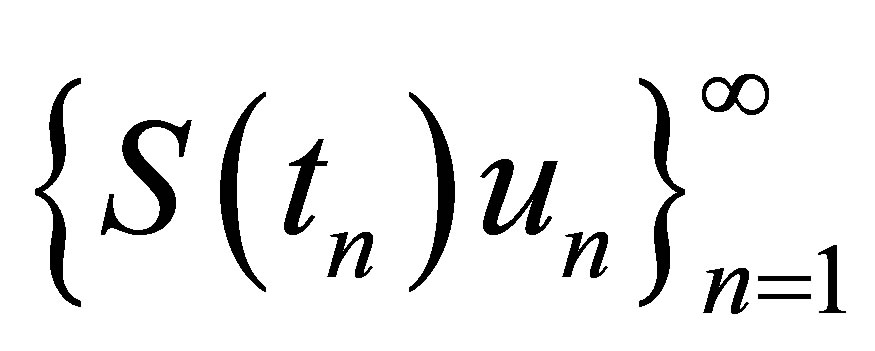 is bounded. Now we can have the sufficiency immediately. This completes the proof of Corollary.
is bounded. Now we can have the sufficiency immediately. This completes the proof of Corollary.
4. Acknowledgements
The author wishes to thank professor Yongluo Cao for his invaluable suggestions and encouragement.