Binding Energy, Root Mean Square Radius and Magnetic Dipole Moment of the Triton Nucleus

Khadija Abdelhassan Kharroube

Faculty of Sciences, Lebanese University, Beirut, Lebanon.

DOI: 10.4236/ojm.2024.142003                

Abstract

The basis functions of the translation invariant shell model are used to construct the ground state nuclear wave functions of ³H. The used residual two-body interactions consist of central, tensor, spin orbit and quadratic spin orbit terms with Gaussian radial dependence. The parameters of these interactions are so chosen in such a way that they represent the long-range attraction and the short-range repulsion of the nucleon-nucleon interactions. These parameters are so chosen to reproduce good agreement between the calculated values of the binding energy, the root mean-square radius, the D-state probability, the magnetic dipole moment and the electric quadrupole moment of the deuteron nucleus. The variation method is then used to calculate the binding energy of triton by varying the oscillator parameter which exists in the nuclear wave function. The obtained nuclear wave functions are then used to calculate the root mean-square radius and the magnetic dipole moment of the triton.

Keywords

Translation Invariant Shell Model, Residual Two-Body Interactions, Nucleon-Nucleon Interactions, Binding Energy, Nuclear Wave Functions

1. Introduction

The nuclear shell model [1] [2] [3] has emerged as a useful description of the atomic nucleus as a many-particle system. Basically, it is perhaps the closest of all nuclear models to being unified, i.e., to describe all properties of all states of all nuclei. Unfortunately, even with the restriction to a shell structure, the number of possible states is often very large and there are few nuclei whose properties can be described without a prohibitive amount of computational labor. Thus, several sub-models of the shell model have been constructed to reduce the number of states and hence also the computational difficulty. These sub-models describe many of the physical structures of states in terms of well-defined quantum numbers.

Simultaneous with the development of the shell model, several collective models have been developed [4] . While collective models consider the coherent motion of nucleons, the shell model takes their independent motion into consideration. Both models are capable of describing qualitative features of nuclear energy levels in their simplest form; however, they are inaccurate in their quantitative descriptions. In the single-particle sub-model, the most extreme version of the shell model is that the orbit of the last odd nucleon defines the properties of states, estimates for electromagnetic transition probabilities and accordingly magnetic moments can be made. Although these estimates are often of the correct order of magnitude, they lack precision. On the other hand, the collective models (one based on the rotation of a nucleus deformed into a non-spherical shape) explained the observed approximate J (J + 1) dependence of bands of energy states of some nuclei and predicted the proportionality of the electromagnetic transitions with the square of a Clebsch-Gordan coefficient [5] . However, the moments of inertia and deformation characteristics must be considered as parameters [6] - [13] . With the development of both types of models, it soon became apparent that they may not be all that different after all. Introducing configuration mixing allows the shell model to go beyond its single-particle features, while the rotational model gains some individual-particle features through the development of the rotating intrinsic state. The core principle underlying the shell model is that the interactions between all the nucleons are governed by an average single-particle potential. We initially assumed that this field was spherically symmetric. In practice, this field is rarely derived from any self-consistent reasoning but rather from the analysis of the physics involved in the problem. Thus, since the nucleons are bound together in a finite restricted region of space, the average field is predicted to be attractive within the nucleus’s confines and to vanish everywhere else. The lowest bound states of an A-nucleons nucleus are created by occupying the lowest energy orbits in the central well, following the Pauli exclusion principle. Each single-particle orbital state can be filled by a maximum of four particles due to the spin and isospin projections of each nucleon: with $\left(m_s,m_t\right)=\left(\frac{1}{2},\frac{1}{2}\right),\left(\frac{1}{2},-\frac{1}{2}\right),\left(-\frac{1}{2},\frac{1}{2}\right)$ , and $\left(-\frac{1}{2}.-\frac{1}{2}\right)$ .

If we are concerned only with the ground state characteristics of certain nucleus, the single-particle orbits of the actual finite potential are like those of an appropriate infinite potential, e.g., the harmonic oscillator well [1] . The advantage of using harmonic oscillator functions is that they are more amenable to mathematical manipulation. We can thus concentrate on the physical many-body aspect of the problem without additional complication in the mathematics. There exist two basic problems in the shell model: first, the introduction of the appropriate residual interaction. Second, finding the configurations of extra core nucleons which form the eigenfunctions.

The residual interaction is supposed to take account of the effects of the nucleon-nucleon interaction which have not been included in the average central field. The effective residual interaction differs from the nuclear force between free nucleons for several reasons. The presence of other nucleons inhibits the final states which may result from any interaction-quite generally, this effect of the Pauli principle, causes the effective residual interaction to have a longer range than the free force [14] . The nucleons polarize other nucleons, so it may be considered that the interaction is taking place between quasi particles which are not real nucleons. Thus, one has some freedom in choosing the effective residual interaction which will lead to eigenfunctions possessing the observed features of the lowest energy levels, e.g., energies, moments, transition probabilities, etc.

The matrix elements of the nuclear Hamiltonian H are negligibly small between states transforming according to different representations of the transformation group, G, of the nucleon motions inside the nucleus. looking for additional groups, it must still be remembered that these must always allow a simultaneous classification according to the rotational group in three dimensions R₃, i.e., states must still have a definite angular momentum [5] .

Nuclear forces are considered largely charge independent. Thus, nuclear states will transform according to the representations of the group SU₂ of two-dimensional special unitary transformations (det = 1) between the two basic isospin components $m_t=\pm \frac{1}{2}$ (i.e., the proton and the neutron). Eigenfunctions can thus be labeled with the representation label the isospin T of SU₂, and functions belonging to the same representation will degenerate in energy. It is also to point out that functions of the same isospin T but different projections M_T belong to nuclei of the same mass number but different charges. Thus, at this stage, all nuclei of the same mass can be treated at the same time if we consider all possible isospin components.

For nuclear forces, the isospin classification is treated as an approximate symmetry. In actual nuclei, however, there exists the Coulomb repulsion between protons, which of course is not charge independent. In light nuclei it is usual to treat Coulomb force as a breaking-symmetry term [1] . Thus, states can still be classified according to isospin, but now states of the same isospin in different nuclei of the same mass number will not degenerate in energy. Isospin then is a broken symmetry.

Another example of an approximate symmetry arises in the Wigner supermultiplet theory [5] . In this case, it is assumed that nuclear forces are not only charge (isospin) independent but also largely spin independent, i.e., the dominant part of the nuclear force operates only in orbital space. Nuclear eigenfunctions can thus be considered to transform according to the representations of the group U₄ of four-dimensional unitary transformations in charge and spin space. Functions which have a definite symmetry according to U₄ also have a definite symmetry according to the group of permutations S_A between the particle numbers of charge-spin states. Remembering that the complete functions representing nuclear states must be totally anti-symmetric with respect to permutations of S_A between particle numbers in the full charge-spin-orbit space, we shall find it perhaps not hard to accept the fact that symmetry with respect to S_A in charge-spin space automatically defines the symmetry in the orbital space. The symmetry of the orbital functions is said to be adjoint to the symmetry of the charge-spin functions. All the orbital functions that transform themselves according to a definite representation of S_A go together with the charge-spin functions of adjoint symmetry to form one totally anti-symmetric nuclear state.

Our exposition will be based on the translation-invariant shell model (TISM), or sometimes called the unitary scheme model (USM) [15] - [23] which is indispensable in considering the clustering effects in the p-shell nuclei. The use of oscillator functions allows us to treat freely the degrees of freedom of the cluster’s internal motion, but we pay for this freedom by having to be content with an incorrect asymptotic behavior of the functions used. This would require some modification of the wave-function tails at low and medium energies (of order 100 - 500 MeV), but it may be acceptable at high energies (E_p ≥ 1 GeV) and at sufficiently high energies of knocked-out clusters, where the volume process dominates.

The ground-state of the triton has total angular momentum $J=\frac{1}{2}$ , isotopic spin $T=\frac{1}{2}$ and even parity, i.e. $\left(J^{\pi },T\right)=\left({\frac{1}{2}}^{+},\frac{1}{2}\right)$ . The nuclear wave function that represents the ground-state of triton can be expanded in series in terms of the basis functions of the TISM. The method of constructing this wave function and calculating the matrix elements of the nucleon-nucleon interaction is well explained in ref. [15] .

Doma et al. [20] , introduced two nucleon-nucleon interactions, each of which contains central, tensor, spin-orbit and quadratic spin-orbit terms with Gaussian radial dependence, which gave results for the ground-state characteristics of the deuteron nucleus in good agreement with the corresponding experimental values and the well-known theoretical results [24] [25] [26] [27] . In the calculations which have been carried out in [20] , the ground-state wave function of the deuteron is expanded in series in terms of the basis functions of the TISM corresponding to even values of the number of quanta of excitations N: $0\le N\le 10$ and orbital angular momentum $\mathcal{l}$ equals 0 for N = 0 and 0, 2 for the other values of N.

In the present paper we derive nucleon-nucleon interactions with one, two, three, and four parameters which fit the ground-state characteristics of deuteron, namely: the binding energy, the D-state probability, the root mean-square radius, the magnetic dipole moment and the electric quadrupole moment. The parameters of the present nucleon-nucleon interactions are chosen in such a way that they can represent the long-range attraction and the short-range repulsion of the nucleon-nucleon interactions. Moreover, we have constructed the ground-state nuclear wave function of triton in terms of the basis functions of the TISM corresponding to even values of N in the range $0\le N\le 10$ . Accordingly, we applied the variation method to calculate the binding energy and the nuclear wave function of triton. Furthermore, we calculated the root mean-square radius and the magnetic dipole moment of the triton nucleus.

2. Classification of States in the TISM

In the nuclear shell model, the spurious states that correspond to the non-zero motion of the center of mass of the whole nucleus, exist and must be eliminated in the calculations. On the other hand, these spurious states, are eliminated from the very beginning in the formulation of the TISM-Hamiltonian by subtracting the center of mass kinetic energy from its Hamiltonian. Hence, the TISM Hamiltonian is free of spurious states. Accordingly, the TISM Hamiltonian describes the mutual motions of A nucleons in a nucleus and is of the form [15] - [19]

$H^{\left(0\right)}={\displaystyle {\sum }_{i=1}^A\left\{\frac{1}{2m}{\left(p_i-\frac{1}{A}{\displaystyle {\sum }_{k=1}^A{p}_k}\right)}^2+\frac{m{\omega }^2}{2}{\left(r_i-\frac{1}{A}{\displaystyle {\sum }_{k=1}^A{r}_k}\right)}^2\right\}}$ , (2.1)

where $r_i$ and $p_i$ are the coordinate and momentum operators of a quasi-particle i, m is the nucleon mass, and $\omega$ is the oscillator frequency. Let us introduce the Jacobi’s transformations [5]

$\begin{array}{l}{x}_{\alpha i}={\displaystyle {\sum }_{k=1}^A{B}_{ik}{\xi }_{\alpha k}},\alpha =1,2,3\\ p_{\alpha i}={\displaystyle {\sum }_{k=1}^A{B}_{ik}{\pi }_{\alpha k}},i,k=1,2,\cdots ,A\end{array}\}$ , (2.2)

where the transformation matrix $B$ satisfies the conditions

$B_{iA}=\frac{1}{\sqrt{A}},{\displaystyle {\sum }_{k=1}^A{B}_{ki}}=\sqrt{A}{\delta }_A^i,i=1,2,\cdots ,A$ . (2.3)

Appling transformations (2.2) to equation (2.1) the result is

$H^{\left(0\right)}={\displaystyle {\sum }_{\alpha =1}^3{\displaystyle {\sum }_{i=1}^{A-1}\left(\frac{1}{2m}{\pi }_{\alpha i}^2+\frac{m{\omega }^2}{2}{\xi }_{\alpha i}^2\right)}}$ . (2.4)

Having the considerations of the second quantization space, we introduce the annihilation and creation oscillator quanta operators as [5] :

$\begin{array}{l}{a}_{\alpha k}^{+}=\sqrt{\frac{m\omega }{2\hslash }}{\xi }_{\alpha k}-\frac{i}{\sqrt{2m\hslash \omega }}{\pi }_{\alpha k},\\ a_{\alpha k}=\sqrt{\frac{m\omega }{2\hslash }}{\xi }_{\alpha k}+\frac{i}{\sqrt{2m\hslash \omega }}{\pi }_{\alpha k}\end{array}$ (2.5)

These operators satisfy the commutation relations

$\left[a_{\alpha i},a_{\beta k}\right]=\left[a_{\alpha i}^{+},a_{\beta k}^{+}\right]=0,\left[a_{\alpha i},a_{\beta k}^{+}\right]={\delta }_{\alpha ,\beta }{\delta }_{i,k}.$ (2.6)

The Hamiltonian operator (2.4) now takes the form:

$H^{\left(0\right)}=\left[{\displaystyle {\sum }_{\alpha =1}^3{\displaystyle {\sum }_{i=1}^{A-1}{a}_{\alpha i}^{+}{a}_{\alpha i}}}+\frac{3}{2}\left(A-1\right)\right]\hslash \omega$ . (2.7)

It can be noticed that it is not so difficult to verify that the Hamiltonian operator (2.7) is invariant with respect to the transformations of the $3\left(A-1\right)$ -dimensional unitary group $U_{3\left(A-1\right)}$ .

The eigenfunctions of the Hamiltonian (2.7) are:

${\phi }_{{\alpha }_1{i}_1,\cdots ,{\alpha }_N{i}_N}=C{a}_{{\alpha }_1{i}_1}^{+}{a}_{{\alpha }_2{i}_2}^{+}\cdots a_{{\alpha }_N{i}_N}^{+}\exp \left\{-\frac{m\omega }{2\hslash }{\displaystyle {\sum }_{\epsilon =1}^3{\displaystyle {\sum }_{i=1}^{A-1}{\xi }_{\epsilon i}^2}}\right\}$ , (2.8)

and the corresponding eigenvalues are given by:

$E_N^{\left(0\right)}=\left[N+\frac{3}{2}\left(A-1\right)\right]\hslash \omega$ . (2.9)

Since the functions (2.8) are symmetric with respect to permutations of any pair of their indices, they may be used as basis for irreducible representation (IR) of a symmetric tensor of the rank N. The Young Scheme {N} is useful for obtaining such IR. It is clear that the dimension of the representation {N} of the group $U_{3\left(A-1\right)}$ is equal to the number of functions ${\phi }_{{\alpha }_1{i}_1,\cdots ,{\alpha }_N{i}_N}$ . The basis functions (2.8) are usually represented by [17] :

$|A\Gamma M_L;{\Gamma }_S{M}_S{M}_T\rangle \equiv |AN\left\{\rho \right\}\left(\nu \right)\alpha \left[f\right]\left(\lambda \mu \right)L{M}_L;\left[\tilde{f}\right]ST{M}_S{M}_T\rangle$ , (2.10)

where $\Gamma$ and ${\Gamma }_S$ are the sets of all orbital and spin-isospin quantum numbers characterizing the states, respectively. The total number of quanta N is the IR of the group $U_{3\left(A-1\right)}$ . The IR of groups U₃ and U_A−1 are set by the same symbols $\left\{\rho \right\}=\left\{{\rho }_1,{\rho }_2,{\rho }_3\right\}$ , where ${\rho }_1\ge {\rho }_2\ge {\rho }_3\ge 0$ are any integers satisfying the requirements ${\rho }_1+{\rho }_2+{\rho }_3=N$ . The symbol (λμ) of the SU₃ symmetry is determined by the relations $\lambda ={\rho }_1-{\rho }_2$ , $\mu ={\rho }_2-{\rho }_3$ , which enables us to find the values of the total orbital angular momentum L, by using Elliott’s rule [5] [28] . According to this rule $L=K,K+1,\cdots ,K+B$ ; $K=C,C-2,\cdots ,1$ or 0 for $K\ne 0$ , $L=B,B-\cdots ,1$ or 0 if $K=0$ , where $C=\min \left(\lambda ,\mu \right)$ and $B=\max \left(\lambda ,\mu \right)$ .

The allowed Young Schemes [f] for the representation $\left\{{\rho }_{\text{1}},{\rho }_2,{\rho }_3\right\}$ of group U_A-1 may be found using the formalism of plethysm, which has been described in detail in [5] [29] . In (2.10) M_L stands for the IR of the group SO₂. The representation ( $\nu$ ) is an IR of the group O_A-1 and [f] is an IR of the symmetric group. The quantum numbers S, M_S are the spin, its projection and T, M_T are the isospin, its projection which are IR of the direct product of the groups $S{U}_2\times S{U}_2$ . Among all the possible Young schemes [f], only those comprising not more than four columns should be selected. If, after that, the values S, T are to be taken for the conjugated Young diagrams $\left[\tilde{f}\right]$ , we shall obtain the total list of the TISM states with given quantum number N.

Wave function with given total quantum numbers $J,M_J,T,M_T$ and parity π can be constructed from the functions (2.10) as follows [15] [17] :

$|A{J}^{\pi }{M}_{J}T{M}_T\rangle ={\displaystyle {\sum }_{\Gamma M_L;{\Gamma }_S{M}_S}{C}_{\Gamma }^{J^{\pi }T}}\left(L{M}_L,S{M}_S|J{M}_J\right)|A\Gamma M_L;{\Gamma }_S{M}_S{M}_T\rangle$ (2.11)

where $C_{\Gamma }^{J^{\pi }T}$ are the state expansion coefficients and $\left(L{M}_L,S{M}_S|J{M}_J\right)$ are Clebsch-Gprdan coefficients (CGC, s).

3. The Supermultiplet Model

The supermultiplet functions can be built, disregarding the internal structure of the orbital wave functions of the nucleus. The supermultiplet model [5] is based on the properties of symmetric group and irreducible tensor spaces of the unitary groups. The method of constructing the supermultiplet wave function of a nucleus is based on that simple position, which follows from the theory of symmetric group, according to the relations:

$\begin{array}{l}\alpha \left(\left[A\right]\times \left[f^{\prime }\right]\to \left[f\right]\right)=\delta \left(\left[f^{\prime }\right],\left[f\right]\right),\\ \alpha \left(\left[1^A\right]\times \left[f^{\prime }\right]\to \left[f\right]\right)=\delta \left(\left[f^{\prime }\right],\left[\tilde{f}\right]\right)\end{array}$ (3.1)

The anti-symmetric representation $\left[1^A\right]$ is contained only in the direct product of the two conjugate representations $\left[f\right]\times \left[\tilde{f}\right]$ . Therefore, the anti-symmetric wave function can be separated into orbital and spin-isospin functions by the following simple form:

$\Psi \left({\Gamma }_0{\Gamma }_s\left(\left[f\right]\left[\tilde{f}\right]\right)\left[1^A\right]\right)={\displaystyle {\sum }_{\mu \tilde{\mu }}{\psi }_{\left[f\right]\mu }\left({\Gamma }_0\right){\psi }_{\left[\tilde{f}\right]\tilde{\mu }}\left({\Gamma }_s\right)C\begin{array}{c}\left[f\right]\\ \mu \end{array}\begin{array}{c}\left[\tilde{f}\right]\\ \tilde{\mu }\end{array}\begin{array}{c}\left[A\right]\\ \left[1^A\right]\end{array}}$ (3.2)

In (3.2), ${\psi }_{\left[f\right]\mu }$ designates orbital and ${\psi }_{\left[\tilde{f}\right]\tilde{\mu }}$ spin-isospin functions characterized by the collections of orbital ${\Gamma }_0$ and spin-isospin ${\Gamma }_s$ quantum numbers and the CGCs of the symmetric group S_A, where

${\Gamma }_0=N\left\{\rho \right\}\left(\nu \right)\left[f\right]L{M}_L$ and ${\Gamma }_s=\left[\tilde{f}\right]ST{M}_s{M}_T$ . (3.3)

The totally anti-symmetric Young Scheme $\left[{\text{1}}^A\right]=\left[\text{11}\cdots \text{1}\right]$ (A-times) is the IR of the group $\mathfrak{J}$ . Since $\left[1^A\right]=\left[f\right]\times \left[\tilde{f}\right]$ therefore, the IR of the group $\mathfrak{J}$ can be reduced to direct product of two unitary groups U_3(A−1) and $U_{4^A}$ corresponding to the orbital and spin-isospin functions. Let $S_A^{\left(S\right)}$ and $S_A^{\left(T\right)}$ be symmetric groups, which transpose respectively spin and isospin coordinates. Then, the spin function is characterized by the diagram

$\left[f_s\right]\equiv \left[\frac{A}{2}+S,\frac{A}{2}-S\right]$ , (3.4)

and the isospin by the diagram

$\left[f_T\right]\equiv \left[\frac{A}{2}+T,\frac{A}{2}-T\right]$ , (3.5)

The corresponding basis is symbolically denoted by

$\Psi ={\psi }_{\left[f_s\right]{\mu }_s}\left(M_s\right){\psi }_{\left[f_T\right]{\mu }_T}\left(M_T\right)$ (3.6)

which is designated through ${\mu }_s$ and ${\mu }_T$ . [f_S] designates both the IR of a group S_A and IR of a group SU₂, which assigns the nucleons.

According to the chain of grpops:

$S_n\supset S_{n-1}\supset \cdots \supset S_2\supset S_1$ . (3.7)

we have

$\left[{\bar{f}}_s\right]=\left[\frac{A-1}{2}+\bar{S},\frac{A-1}{2}-\bar{S}\right]$

$\left[{\overline{\stackrel{¯}{f}}}_s\right]=\left[\frac{A-2}{2}+\overline{\stackrel{¯}{S}},\frac{A-2}{2}-\overline{\stackrel{¯}{S}}\right]$ , etc. (3.8)

Obviously, the spin-isospin function of the nucleus can be built via the binding of ideas [f_S] and [f_T] by the CGC,s of the symmetric group S_A, so that

${\Psi }_{\left[\tilde{f}\right]\tilde{\mu }}\left({\Gamma }_s\right)={\displaystyle {\sum }_{{\mu }_s{\mu }_T}{\psi }_{\left[f_s\right]{\mu }_s}\left(M_S\right){\psi }_{\left[f_T\right]{\mu }_T}\left(M_T\right)C\begin{array}{c}\left[f_S\right]\\ {\mu }_S\end{array}\begin{array}{c}\left[f_T\right]\\ {\mu }_T\end{array}\begin{array}{c}\tilde{\alpha }\left[\tilde{f}\right]\\ \tilde{\mu }\end{array}}$ (3.9)

With the aid of formulas (3.2) and (3.9) we actually achieved the construction of the supermultiplet wave function of the nucleus, and for this purpose it is sufficiently enough to use only two types of CGC,s of the group S_A satisfying the relations

$\left[f\right]\times \left[\tilde{f}\right]\to \left[1^A\right]$ , (3.10)

and

$\left[f_S\right]\times \left[f_T\right]\to \tilde{\alpha }\left[\tilde{f}\right]$ (3.11)

Subsequently, the supermultiplet wave function of the nucleus is designated by:

$\Psi =\Psi \left({\Gamma }_0\left(\left[f\right]\left(\left[f_S\right]\left[f_T\right]\right)\tilde{\alpha }\left[\tilde{f}\right]\right)\left[1^A\right]M_S{M}_T\right)$ (3.12)

Let us further consider that the states of nuclei must be described by quantum number J of the total angular momentum $J=L+S$ . Hence, it follows that the collection Γ₀ must include the quantum number of the total orbital angular momentum L and of its projection M_L. Therefore, if we replaced Γ₀ in (3.12) with new collection ${\Gamma }_{0}L{M}_L$ and to connect the momenta L and S in J, then the supermultiplet wave function of the nucleus with the most complete characteristic takes the following form:

$\Psi \left({\Gamma }_0\pi \left(\left[f\right]\left(\left[f_S\right]\left[f_T\right]\right)\tilde{\alpha }\left[\tilde{f}\right]\right)\left[1^A\right]J{M}_J{M}_T\right)$ (3.13)

In (3.13), the quantum number π, determins the parity of the orbital wave function of the nucleus.

We find $\left[\tilde{f}\right]$ , together with the representation of a group S_A, also does designate IR of a group SU₄, and this IR is given in the chain of groups: $S{U}_4\supset S{U}_2\times S{U}_2,$ which in turn does lead to quantum numbers S, M_S and T, M_T. This sense acquires and bringing $\left[f_S\right]\times \left[f_T\right]\to \tilde{\alpha }\left[\tilde{f}\right]$ , which with the use of transformation properties of group SU₄ should be written in the form:

$\left[\tilde{f}\right]={\displaystyle {\sum }_{\left[f_S\right]\left[f_T\right]}\tilde{\alpha }}\left(\left[\tilde{f}\right]\supset \left[f_S\right]\left[f_T\right]\right)\left[f_S\right]\left[f_T\right]$ , (3.14)

here $\tilde{\alpha }$ is the number of repetitions of identical $\left[f_S\right]$ , $\left[f_T\right]$ in $\left[\tilde{f}\right]$ . It can be, in abbreviated form, designated by:

$\left[\tilde{f}\right]={\displaystyle {\sum }_{ST}\tilde{\alpha }}\left(\left[\tilde{f}\right]\supset ST\right)\left[S,T\right].$ (3.15)

All possible values of S and T belonging to the diagram $\left[\tilde{f}\right]$ are obtained in the brackets [S, T].

4. The Nuclear Hamiltonian and the Nucleon-Nucleon Interactions

The internal Hamiltonian of a nucleus consisting of A nucleons can be written in terms of the relative coordinates of the nucleons, in the form [15] [17] [21]

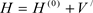$H=H^{\left(0\right)}+V^{(\text{'})}$ (4.1)

where

$H^{\left(0\right)}=\frac{1}{A}{\displaystyle {\sum }_{i=1}^A\left[\frac{{\left(p_i-p_j\right)}^2}{2m}+\frac{1}{2}m{\omega }^2{\left(r_i-r_j\right)}^2\right]}$ , (4.2)

is the TISM-Hamiltonian and

$V^{(\text{'})}={\displaystyle {\sum }_{1=i<j}^A\left[V\left(\left|r_i-r_j\right|\right)-\frac{m{\omega }^2}{2A}{\left(r_i-r_j\right)}^2\right]}$ . (4.3)

is the two-body residual interaction.

For the two-nucleon states with orbital angular momentum $\mathcal{l}$ , spin momentum s and isotopic spin t, our two-nucleon interaction has the form [20] :

$V\left(r\right)={}^{\text{ts}}X\left\{V_C\left(r\right)+V_T\left(r\right)S_{12}+V_{LS}\left(r\right)\mathcal{l}\cdot s+V_{LL}\left(r\right)L_{12}\right\}$ (4.4)

The central, tensor, spin-orbit and quadratic spin-orbit terms are standard. The operator ${}^{\text{ts}}X$ has the form

${}^{\text{ts}}X=C_W+{\left(-1\right)}^{s+t+1}{C}_M{\left(-1\right)}^{s+1}{C}_B+{\left(-1\right)}^{t+1}{C}_H$ , (4.5)

where $C_W,C_M,C_B$ and $C_H$ are the Wigner, the Majorana, the Bartlett and the Heisenberg exchange constants, respectively. Each term of the interaction is expressed as a sum of Gaussian functions in the form

$V_{\alpha }\left(r\right)={\displaystyle {\sum }_{k=1}^{2,3,4}{V}_{\alpha k}{\text{e}}^{-r^2/r_{\alpha k}^2}}$ (4.6)

where $\alpha =C,T,LS$ and LL. Two sets of values are considered for the exchange constants, namely: C_W = 0.1333, C_M = −0.9333, C_B = −0.4667, C_H = −0.2667, which are known as the Rosenfeld constants, and belong to the symmetric case, and C_W = −0.41, C_M = −0.41, C_B = −0.09, C_H = 0.09 which belong to the Serber case. For the two-particle triplet-even state (t = 0, s = 1), which is the case for the ground-state of the deuteron nucleus, and from the normalization condition of the exchange constants, the operator ^tsX equals −1 for both of the symmetric and the Serber cases so that the two types of the exchange constants will produce the same results for the ground-state characteristics of deuteron.

Our realistic two-nucleon interactions underbind the ground-state energies of the A = 2, 3, 4 nuclei. It appears that a simple two-pion exchange three-nucleon interaction cannot give attraction in A = 3 nuclei [19] [22] .

The matrix elements of the residual two-body interaction $V^{\prime }$ with respect to the basis (2.11) are given by [15] [17] [18] [19] [22] :

$\begin{array}{l}\langle A{J}^{\pi }{M}_{J}T{M}_T|V^{\prime }|A{J}^{\pi }{M}_{J}T{M}_T\rangle \\ =\frac{A\left(A-1\right)}{2}{\displaystyle {\sum }_{\bar{\Gamma }\epsilon \mathcal{l}sjt{\epsilon }^{\prime }{\mathcal{l}}^{\prime }}{C}_{\Gamma }^{J^{\pi }T}{C}_{{\Gamma }^{\prime }}^{J^{\pi }T}}\left(L{M}_L,S{M}_S|J{M}_J\right)\left(L^{\prime }{M^{\prime }}_L,S^{\prime }{M^{\prime }}_S|J{M}_J\right)\\ \times \langle A\Gamma JT|A-2\bar{\Gamma };2\epsilon \mathcal{l}sjt\rangle \times \langle A\Gamma JT|A-2\bar{\Gamma };2{\epsilon }^{\prime }{\mathcal{l}}^{\prime }sjt\rangle \left(\begin{array}{ccc}\bar{L}& \bar{S}& \bar{J}\\ \mathcal{l}& s& j\\ L& S& J\end{array}\right)\\ \times \left(\begin{array}{ccc}\bar{L}& \bar{S}& \bar{J}\\ {\mathcal{l}}^{\prime }& s& j\\ L^{\prime }& S^{\prime }& J\end{array}\right)\langle \left(\epsilon \mathcal{l}s\right)jt|{V^{\prime }}_{A-1,A}|\left({\epsilon }^{\prime }{\mathcal{l}}^{\prime }s\right)jt\rangle \end{array}$ (4.7)

Here $\bar{\Gamma }$ stands for the set of all orbital and spin-isospin quantum numbers of the A-2 particles. The number of quanta for the two-particle wave function $\epsilon =2n+\mathcal{l}$ , in which n is the radial quantum number of the inter-particle distance joining the last pair. $\mathcal{l}$ , s, j and t are the orbital, the spin, the total spin and the isospin quantum numbers of the last pair. In Equation (4.7) $\langle A\Gamma JT|A-2\bar{\Gamma };2\epsilon \mathcal{l}sjt\rangle$ are the two-particle total fractional parentage coefficients which are products of orbital and spin-isospin coefficients [19] [23] . The last elements in Equation (4.7) are the two-particle matrix elements of the residual interaction where: ${V^{\prime }}_{A-1,A}=V^{\prime }\left(\left|r_{A-1}-r_A\right|\right)$ and $\left(\begin{array}{ccc}{j}_1& j_2& j_{12}\\ j_3& j_4& j_{34}\\ j_{13}& j_{24}& J\end{array}\right)$ are the normalized 9j-symbols.

Thus, the energy matrices can be constructed according to Equations (2.9) and (4.7) for the different states of a nucleus with mass number A, for each residual interaction, as functions of the oscillator parameter $\hslash \omega$ . These matrices are diagonalized with respect to $\hslash \omega$ which is allowed to vary in a wide range of values, $8\le \hslash \omega \le 30$ MeV, in order to obtain the best fit to the spectrum of this nucleus or to the best fit to the binding energy, in the case where the nucleus has no excited states. Hence, the energy eigenvalues and eigenfunctions of the ground state of this nucleus are obtained for each considered potential.

5. The Root Mean-Square Radius and the Magnetic Dipole Moment

The ground-state nuclear wave function, which is obtained as a consequence of the diagonalization of the ground-state energy matrix, is used to calculate the root mean-square radius from the well-known formula [21] [22] [23]

$\begin{array}{c}R=[r_p^2+\frac{A-1}{A\left[m\omega /\hslash \right]}\times {\displaystyle {\sum }_{\Gamma {\Gamma }^{\prime }\bar{\Gamma }\epsilon \mathcal{l}sjt{\epsilon }^{″}{\mathcal{l}}^{\prime }}{C}_{\Gamma }^{J^{\pi }T}{C}_{{\Gamma }^{\prime }}^{J^{\pi }T}}\times \langle A\Gamma JT|A-2\bar{\Gamma };2\epsilon \mathcal{l}sjt\rangle \\ \times \langle A\Gamma JT|A-2\bar{\Gamma };2\epsilon \mathcal{l}sjt\rangle \{\left(\epsilon +\frac{3}{2}\right){\delta }_{{\epsilon }^{\prime },\epsilon }-\frac{1}{2}\sqrt{\left(\epsilon -\mathcal{l}+2\right)\left(\epsilon +\mathcal{l}+3\right)}{\delta }_{{\epsilon }^{\prime },\epsilon +2}\\ -{\frac{1}{2}\sqrt{\left(\epsilon -\mathcal{l}\right)\left(\epsilon +\mathcal{l}+1\right)}{\delta }_{{\epsilon }^{\prime },\epsilon +2}\}{\delta }_{{\mathcal{l}}^{\prime },\mathcal{l}}{\delta }_{\left({\nu }^{\prime }\right),\left(\nu \right)}{\delta }_{\left[f^{\prime }\right],\left[f\right]}{\delta }_{\text{'}{S}^{\prime },S}]}^{\frac{1}{2}},\end{array}$ (5.1)

where $r_p=0.85$ fm is the proton radius and m is the nucleon mass.

The magnetic dipole moment operator $\hat{\mu }$ for a nucleus consisting of A nucleons is defined as the expectation value of the operator [19] [21]

$\hat{\mu }={\hat{\mu }}_o+{\hat{\mu }}_{\sigma }$ , (5.2)

where

${\hat{\mu }}_o=\frac{1}{2}{\displaystyle {\sum }_{i=1}^A\left(1-2t_{oi}\right){\mathcal{l}}_{oi}}$ (5.3)

and

${\hat{\mu }}_{\sigma }={\displaystyle {\sum }_{i=1}^A\left[\left({\mu }_p+{\mu }_n\right)+2\left({\mu }_p-{\mu }_n\right)t_{oi}\right]s_{oi}}$ (5.4)

In Equations (5.3) and (5.4) ${\mathcal{l}}_{0i}$ , $s_{0i}$ and $t_{0i}$ are the z-components of the orbital, the spin, and the isospin momenta of the i^th-nucleon, respectively. ${\mu }_p$ and ${\mu }_n$ are the proton and the neutron magnetic moments, respectively. The matrix elements of the orbital operator ${\hat{\mu }}_o$ and the spin-isospin operator ${\hat{\mu }}_{\sigma }$ are calculated in a nuclear state having $M_J=J$ . The method of calculating these matrix elements are given by applying the supermultiplet model of the nucleus [21] .

6. Results and Conclusions

In the present paper, we introduce four new nucleon-nucleon interactions in the form given by Equations (4.3) - (4.6). The first is a one-parameter potential, the second is a two-parameter potential, the third is a three-parameter potential, and the fourth is a four-parameter potential. In Tables 1-4, we present the parameters of our four interactions, for which the calculated values of the deuteron characteristics are in good agreement with the corresponding experimental values. The values of the depth parameters V are given in MeV and the values of the range parameters r are given in fm.

In Table 5, we present the calculated deuteron characteristics by using the four new interactions. The second column is for the deuteron binding energy (DBE), the third for the deuteron root-mean square radius (DRM), the fourth for the deuteron D-state probability (DDSP), the fifth for the deuteron magnetic

Table 1. Depth and range parameters for the one-parameter potential (pot-I).

Table 2. Depth and range parameters for the two-parameters potential (pot-II).

Table 3. Depth and range parameters for the three-parameters potential (pot-III).

Table 4. Depth and range parameters for the four-parameters potential (pot-IV).

Table 5. Deuteron characteristics by using the four nucleon-nucleon interactions.

dipole moment (DMD), and the sixth for the deuteron electric quadrupole moment (DQM). The experimental values [24] [25] [26] [27] are also given in the last row of this table.

For the triton nucleus, our total nuclear wave function is obtained by using the TISM wave functions given by Equation (2.11) with a number of quanta of excitation N = 0, 2, 4, 6, 8, and 10, total spin $S=\frac{1}{2}$ , total isospin $T=\frac{1}{2}$ , and even parity. Accordingly, we applied the variational method to calculate the triton ground state energ eigenvalue and eigenfunction, by varying the oscillator parameter, $\hslash \omega$ in a wide range of values: $8\le \hslash \omega \le 20$ MeV to obtain the best values. The obtained nuclear wave function is used to calculate the triton binding energy (B.E.), root mean square radius (R), and magnetic dipole moment (μ).

In Figure 1, we present the variation of the binding energy of triton with respect to the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

In Figure 2, we present the variation of the root mean square radius of triton as a function of the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

In Figure 3, we present the variation of the magnetic dipole moment of triton as a function of the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

In Table 6, we present the triton binding energy (B.E.), root mean-square radius (R), magnetic dipole moment (μ), and oscillator parameter ( $\hslash \omega$ ), by using the four potentials.

Table 7 presents all of the energy expectation values, as well as point-particle

Figure 1. Binding energy of triton as function of the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

Figure 2. Root mean square radius of triton as function of the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

Figure 3. Magnetic dipole moment of triton as function of the oscillator parameter $\hslash \omega$ by using the fourth potential Pot-IV.

Table 6. Triton results using the four potentials. The experimental values are also given.

Table 7. Role of the different operators in the triton binding energy for the fourth potential Pot-IV.

root mean square radii of the neutron and proton density by using the fourth potential. In this Table, V gives the total nucleon-nucleon potential energy, and T is the total kinetic energy.

As we see, the fourth potential gives the best results for both of the deuteron characteristics and the triton characteristics which are in good agreement with the corresponding experimental values rather than the other three potentials.

The results of ref. [29] shown that the three-body interaction can explain the binding energy and the dip in the proton distribution of the ³He nucleus. The effect of the spin-isospin dependence of the three-nucleon interaction can bring the theory of this nucleus closer to the experiment. However, the inclusion of the three-body force in the model Hamiltonian of the triton nucleus can improve the ground-state characteristics of the triton by no more than 1.0% [30] .

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	De-Shalit, A. and Talmi, I. (2013) Nuclear Shell Theory. Academic Press, New York.
[2]	Bargman, V. and Moshinsky, M. (1961) Group Theory of Harmonic Oscillators. I. The Collective Modes. Nuclear Physics, 18, 697-712. https://doi.org/10.1016/0029-5582(60)90438-7
[3]	Kramer, P. and Moshinsky, M. (1966) Group Theory of Harmonic Oscillators (III). States with Permutational Symmetry. Nuclear Physics, 82, 241-274. https://doi.org/10.1016/0029-5582(66)90001-0
[4]	Ring, P. and Schuck, P. (1980) The Nuclear Many-Body Problem. Springer Verlag, New York. https://doi.org/10.1007/978-3-642-61852-9
[5]	Vanagas, V.V. (1971) Algebraic Methods in Nuclear Theory. Mintis, Vilnius.
[6]	Doma, S.B. (2002) Single Particle Schrödinger Fluid and Moments of Inertia of Deformed Nuclei. Journal High Energy Physics and Nuclear Physics, 26, 836-842.
[7]	Doma, S.B., Kharroube, K.A., Tefiha, A.D. and El-Gendy, H.S. (2011) The Deformation Structure of the Even-Even p-and sd Shell Nuclei. Alexandria Journal of Physics, 1, 1-13.
[8]	Doma, S.B. (2003) The Single Particle Schrodinger Fluid and Moments of Inertia of Deformed Nuclei. Bulgarian Journal of Physics, 30, 117-124.
[9]	Doma, S.B. and Amin, M.M. (2009) Single Particle Schrödinger Fluid and Moments of Inertia of the Even-Even Uranium Isotopes. The Open Applied Mathematics Journal, 3, 1-6. https://doi.org/10.2174/1874114200903010001
[10]	Doma, S.B. and El-Gendy, H.S. (2018) A Nuclear Phenomenological Study of the Even-Even Thorium Isotopes. International Journal of Modern Physics E, 27, Article 1850040. https://doi.org/10.1142/S0218301318500404
[11]	Hammad, M.M., Yahia, M.M., Motaweh, H.A. and Doma, S.B. (2020) Critical Potentials and Fluctuations Phenomena with Quartic, Sextic, and Octic Anharmonic Oscillator Potentials. Nuclear Physics A, 1004, Article 122036. https://doi.org/10.1016/j.nuclphysa.2020.122036
[12]	Hammad, M.M., Shetawy, H.E., Aly, A.A. and Doma, S.B. (2019) Nuclear Supersymmetry and Dual Algebraic Structures. Physica Scripta, 94, Article 105207. https://doi.org/10.1088/1402-4896/ab2442
[13]	Hammad, M.M., Yaqut, A.S.H., Abdel-Khalek, M.A. and Doma, S.B. (2021) Analytical Study of Conformable Fractional Bohr Hamiltonian with Kratzer Potential. Nuclear Physics A, 1015, Article 122307. https://doi.org/10.1016/j.nuclphysa.2021.122307
[14]	Blatt, J.M. and Weisskopf, V.F. (1979) Theoretical Nuclear Physics. Springer-Verlag, New York. https://doi.org/10.1007/978-1-4612-9959-2
[15]	Doma, S.B. (2003) Study of Nuclei with A = 5 on the Basis of the Unitary Scheme Model. International Journal of Modern Physics E, 12, 421-429. https://doi.org/10.1142/S021830130300134X
[16]	Doma, S.B. and El-Zebidy, A.F.M. (2005) Cluster-Cluster Potentials for the Lithium Nuclei. International Journal of Modern Physics E, 14, 189-195. https://doi.org/10.1142/S0218301305002989
[17]	Doma, S.B. (1985) Unitary Scheme Model Study of 4He with the Gogny, Pires and Detourreil Interaction. Helvetica Physica Acta, 58, 1072-1077.
[18]	Doma, S.B., El-Zebidy, A.F.M. and Abdel-Khalik, M.A. (2006) The Mean Lifetime of the β-Decay and the Nuclear Magnetic Dipole Moment for Nuclei with A = 7. Journal of Physics G: Nuclear and Particle Physics, 34, 27. https://doi.org/10.1088/0954-3899/34/1/002
[19]	Doma, S.B. and El-Gendy, H.S. (2018) Unitary Scheme Model Calculations of the Ground and Excited State Characteristics of 3H and 4He. Journal of Physics Communications, 2, Article 065005. https://doi.org/10.1088/2399-6528/aac79f
[20]	Doma, S.B., El-Nohy, N.A. and Gharib, K.K. (1996) The Ground-State Characteristics of Deuteron Using Gaussian Potentials. Helvetica Physica Acta, 69, 90-104.
[21]	Doma, S.B., El-Gendy, H.S. and Hammad, M.M. (2020) Large Basis Unitary Scheme Model Calculations for the Mirror Nuclei with A = 7. Chinese Journal of Physics, 63, 21-35. https://doi.org/10.1016/j.cjph.2019.10.026
[22]	Doma, S.B. (2002) Ground State Characteristics of the Light Nuclei with A ≤ 6 on the Basis of the Translation Invariant Shell Model by Using Nucleon-Nucleon Interactions. Chinese Physics C, 26, 941-948.
[23]	Doma, S.B. (2012) Unitary Scheme Model Calculations of A = 6 Nuclei with Realistic Interactions. International Journal of Modern Physics E, 21, Article 1250077.
[24]	Ericson, T.E.O. and Rosa-Clot, M. (1983) The Deuteron Asymptotic D-State as a Probe of the Nucleon-Nucleon Force. Nuclear Physics A, 405, 497-533. https://doi.org/10.1016/0375-9474(83)90516-X
[25]	Ericson, T.E.O. (1984) The Deuteron Properties. Nuclear Physics A, 416, 281-298. https://doi.org/10.1016/0375-9474(84)90467-6
[26]	Rodning, N.L., Madison, W.U. and Knutspn, L.D. (1990) Asymptotic D-State to S-State Ratio of the Deuteron. Physical Review C, 41, 898-909. https://doi.org/10.1103/PhysRevC.41.898
[27]	Samuel, S.M.W. (1990) Introductory Nuclear Physics. Prentice Hall, Englewood Cliffs, NJ.
[28]	Zheng, D.C., Vary, J.P. and Barrett, B.R. (1994) Large-Space Shell-Model Calculations for Light Nuclei. Physical Review C, 50, 2841-2849. https://doi.org/10.1103/PhysRevC.50.2841
[29]	Carlson, J. and Pandharipande, V.R. (1981) A Study of Three-Nucleon Interaction in Three-and Four-Body Nuclei. Nuclear Physics A, 371, 301-317. https://doi.org/10.1016/0375-9474(81)90069-5
[30]	Friar, J.L. (1987) Results of Recent Calculations Using Realistic Potentials, Few-Body Problems in Particle, Nuclear, Atomic, and Molecular Physics. Proceedings of the XIth-European Conference on Few-Body Physics, Fontevraud, 31 September-5 August 1987, 51-63. https://doi.org/10.1007/978-3-7091-8956-6_4