1. Introduction
In recent years, the study of 3-Lie algebra has been paid much attention because of its wide application in mathematics and physics. 3-Lie algebra is a special form of n-Lie algebra, which is an algebraic system with ternary linearly oblique symmetric multiplication table satisfying the generalized Jacobi equation [1]. 3-Lie algebra has extremely profound and rich algebraic and analytical structure. In this paper, the extension problem of 3-Lie algebra is studied on the basis of the existing research. Firstly, we define the unary extended 3-Lie algebra for a known Lie algebra L by one-dimensional extension, and study its properties. Secondly, for two known 3-Lie algebras H, M, the
-extension of H through M is defined, and the
-extension of H through M is given as a necessary and sufficient condition for the 3-Lie algebra. Finally, the structure and properties of this extended 3-Lie algebra are discussed. Thus, it lays a foundation for the further study of the properties of the derivatives of two kinds of 3-Lie algebras.
2. Fundamental Notions
Firstly, the basic knowledge [1] - [9] to be used in this paper is given.
Definition 2.1 Let A be a vector space over a domain F and have a 3-element linear operation
, satisfied for arbitrary,
, (1)
is called 3-Lie algebra. Without confusion, A is called 3-Lie algebra for short.
Definition 2.2 Let A be a 3-Lie algebra, and D be a linear transformation ofA, if this equation is satisfied
,
(2)
Then D is the derivative of A, and the set of derivatives is denoted by
. It is easy to prove that
is a subalgebra of the general linear Lie algebra
.
The map
,
for
is called the left multiplication defined by elements
. Obviously the left multiplication is the derivative. The linear combination of the left multiplication is called the inner derivative, denoted by
.
Let B be a subspace ofA, and if
, then B be a subalgebra (ideal) of A. And if
, then B is called a Abel subalgebra
(Abel ideal). In particular, the subalgebra spanned by
is called the derivative algebra of A, denoted by
. If
, then A is called Abel algebra. If an ideal I of A is a Abel subalgebra but not an Abel ideal, that is
, but
, then I is called an hypo-abelian ideal.
The ideal I of a 3-Lie algebra A is called s-solvable,
, if
for some
, where
,
is defined as
. Where 2-solvable is also called solvable, and
is abbreviated as
.
An ideal I of a 3-Lie algebra A is called nilpotent if
for some
, where
and
.
The center of A is denoted by
. Obviously
is the Abel ideal of A.
Let A is a 3-Lie algebra over the field F, V is a vector space,
is a linear mapping, if
satisfies for any
(3)
(4)
Then
is called the representation of A (or
is A-module).
Lemma 2.1 Let A is a 3-Lie algebra over the field F, V is a vector space,
is a linear mapping. If
is an A-module, then for any
, the following equation is true:
, (5)
(6)
3. The Unary Extension 3-Lie Algebra of Lie Algebras
Definition 3.1 Let
be a Lie algebra over a field F, let
, and
. Linear operation
for all
that satisfy the following multiplication table:
. (7)
Then A is called the unary extension of Lie algebra L. If
is a 3-Lie algebra, then
is a unary extension 3-Lie algebra of the Lie algebra L.
Lemma 3.1 let L be a Lie algebra over a field F. If let
,
and the multiplication of is defined by (7), then A is a 3-Lie algebra, and for positive integers m, the following equation holds
Proof: By multiplication (7), direct calculation A is 3-Lie algebra. Due to the
,
,
Assume
, then
.
similarly,
and
. The conclusion is proved.
Theorem 3.1 Let L be a Lie algebra on the field F and
be a unary extension 3-Lie algebra, where
and
, then
1) A is 2-solvable if and only if L is a solvable Lie algebra.
2) A is nilpotent if and only if L is a nilpotent Lie algebra.
3) A is 3-solvable.
4)
.
Proof: According to lemma 3.1, (1), (2) and (3) can be obtained directly. It is proved below that (4) is true. If
, then
and
. If
, then exists
such that
. For any
,
,
, because of
, therefore
.And because
, so
. Therefore
. Obviously, the conclusion of
is true.
Theorem 3.2 Let L be a Lie algebra on the field F and I be a subspace ofL:
1) I is an ideal of A if and only if I is an ideal of L.
2) Let
, then J is ideal of A if and only if
.
3) If
, then for positive integers m,
. If I is a solvable ideal of L, then J is a 2-solvable ideal of A.
4) If L is a simple Lie algebra, then L is hypo-abelian ideal of A.
Proof: From
, we can get (1). From Equation (7),
,
So
if and only if
. That means (2) is true.
If I is the ideal of L and
, then
,
,
Assuming
is true, then
.
Therefore (3) holds. If L is a simple Lie algebra, then L is ideal of A, and
,
. Therefore, L is hypo-abelian ideal of A. That’s the end of the argument.
4.
-Extension of 3-Lie Algebras
Definition 4.1 Let
and
be two 3-Lie algebras over the field F,
, and
,
,
is linear mappings. Define a linear operation
, for any
,
that satisfies the multiplication table:
,
(8)
,
.
Then
is called the
-extension of H through M. If
is a 3-Lie algebra, then
is
-extension algebra of 3-Lie algebra. If
, then A is called
-extension of H through M, and
denoted as
. For convenience, we will abbreviate
and
as
and
as
.
Lemma 4.1 Let
and
be two 3-Lie algebras over the field F, and A be the
-extension of H through M, and for all
satisfy
(9)
Then Equation (6) is true if and only if the following equation
(10)
Proof: From Equation (9), we can get
So Equation (10) holds. On the other hand, if
Through Equation (9), it can be concluded that Equation (6) holds.
Lemma 4.2. Let A be the
-extension of H through M, for all
,
satisfies
(11)
There are
(12)
Proof: From Equation (11) and the
is derivative of H, we can get
so
Namely
Using Equation (11) again, Equation (12) can be obtained.
Lemma 4.3. Let A be the
-extension of H through M. If for all
, 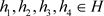 satisfies
satisfies
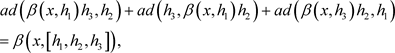 (13)
(13)
Then
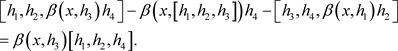 (14)
(14)
Proof: According to Equation (13),
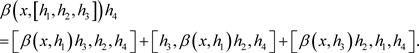
Because of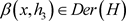 , therefore
, therefore
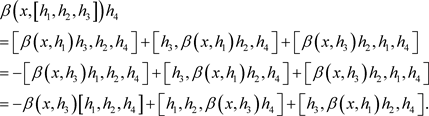
Hence, Equation (14) holds.
Theorem 4.1. Let A be the 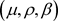 -extension of H through M, then A is a 3-Lie algebra if and only if for any
-extension of H through M, then A is a 3-Lie algebra if and only if for any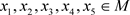 ,
, 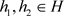 , Equations (6), (9), (11), (13) and the following are true,
, Equations (6), (9), (11), (13) and the following are true,
 (15)
(15)
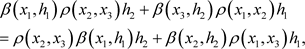 (16)
(16)
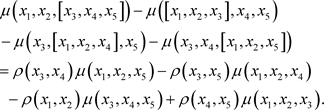 (17)
(17)
Proof: If A is a 3-Lie algebra, the Equations (11), (15), (16) and (17) are obtained from the Equations (1). The following proves that Equations (6), (9) and (13) are true.
For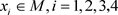 ,
, 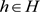 , according to (8),
, according to (8),
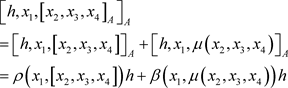
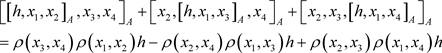
As a result,

In the above formula,  is replaced by
is replaced by![]() , and Equation (9) can be obtained.
, and Equation (9) can be obtained.
Because, ![]()
![]()
So Equation (10) holds. Equation (6) is obtained from lemma 4.1.
For arbitrary![]() ,
, ![]() , it can be known from (8) that,
, it can be known from (8) that,
![]()
![]()
As a result,
![]()
Because of![]() , therefore
, therefore
![]()
Equation (13) holds.
Conversely, to prove that A is a 3-Lie algebra, it is only necessary to prove that (8) satisfies Equation (1).
Case 1. For all![]() , known by (8)
, known by (8)
![]()
![]()
From Equation (17), we can get
![]()
Case 2. For all![]() ,
, ![]() , know from (8)
, know from (8)
![]()
![]()
In Equation (9), by ![]() substitution for
substitution for![]() , we can get
, we can get
![]()
As a result,
![]()
Due to the![]() ,
,
![]()
Through lemma 4.1 and Equation (9), we can get
![]()
![]()
According to Equations (6) and (10),
![]()
Case 3. For all![]() ,
, ![]() , it is obtained from Equations (15), (16)
, it is obtained from Equations (15), (16)
![]()
![]()
Because![]() ,
,
![]()
Through the direct calculation of Equations (15) and (16),
![]()
![]()
![]()
As a result,
![]()
Case 4. For all![]() ,
, ![]() , due to the
, due to the![]() , it can be concluded from Equation (11) that,
, it can be concluded from Equation (11) that,
![]()
![]()
Because of![]() ,
,
Then
![]()
According to lemma 4.2,
![]()
![]()
Using Equation (11) again, we can get
![]()
namely
![]() .
.
Case 5. For all![]() ,
, ![]() , because
, because![]() , through Equation (13),
, through Equation (13),
![]()
![]()
To sum up, (8) satisfies Equation (1). The conclusion is proved.
Theorem 4.2 Let ![]() be the
be the ![]() -extension of 3-Lie algebra H through M. So
-extension of 3-Lie algebra H through M. So ![]() is M-module if and only if
is M-module if and only if![]() .
.
Proof: If![]() , obviously
, obviously ![]() is an M-module.
is an M-module.
On the other hand, to any![]() , by theorem 4.1 and Equation (9), (10),
, by theorem 4.1 and Equation (9), (10),
![]()
![]()
As a result,
![]()
![]()
![]()
According to Equation (9),![]() . And the theorem is proved.
. And the theorem is proved.
Theorem 4.3 Let ![]() be the
be the ![]() -extension of 3-Lie algebra H through M and
-extension of 3-Lie algebra H through M and ![]() be an M-module. So A is a 3-Lie algebra if and only if
be an M-module. So A is a 3-Lie algebra if and only if![]() ,
, ![]() and Equation (17) is true.
and Equation (17) is true.
Proof: If A is a 3-Lie algebra, Equation (17) holds by theorem 4.1. Since![]() is M-module, then
is M-module, then![]() . And
. And![]() ,
, ![]() can be obtained by Equations (9) and (13). Conversely, from theorems 4.1 and 4.2, A is a 3-Lie algebra.
can be obtained by Equations (9) and (13). Conversely, from theorems 4.1 and 4.2, A is a 3-Lie algebra.
The above conclusions about 3-Lie algebras will be helpful for further study of their derivation algebras.
Funding
Science and Technology Research Project of Higher Education Department of Hebei Province (Z2015009).