1. Introduction
The concept of projective change between two Finsler spaces has been studied by many geometers [1] - [6]. An interesting result concerned with the theory of projective change was given by Rapscak [7]. He proved necessary and sufficient conditions for projective change. S. Bacso and M. Matsumoto [8] discussed the projective change between Finsler spaces with
-metric. H. S. Park and Y. Lee have studied on projective changes between a Finsler space with
- metric and the associated Riemannian metric.
In Riemannian geometry, two Riemannian metrics
and
on a manifold M are projectively related if and only if their spray coefficients have the relation
, where
is a scalar function on M and
. In Finsler geometry, two Finsler metrics F and
on a manifold M are called projectively related if
, where
and
are the geodesic coefficients of F and
, respectively and
is a scalar function on the slit tangent bundle
.
In [9], we introduced the generalized
-metric
(1.1)
where
is a Riemannian metric,
is a 1-form.
We know from [4], that two Finsler metrics F and
are projectively related if and only if their spray coefficients have the following relation:
(1.2)
where
is a scalar function on
and homogeneous of degree one in y.
Also, from [1] we know that a Finsler metric is called a projectively flat metric if it is projectively related to a Minkowskian metric. From [4], we know that the Randers metric
is projectively flat if and only if
is projectively flat and
is closed.
The purpose of the present paper is to continue the study on the generalized
-metric
and to investigate the locally projective flatness. Also, the projective change between between generalized
-metric
and Randers metric
, where
and
are
two Riemannian metrics,
and
are 1-forms. Further, we characterized such projective change. Precisely, we have the following
Theorem 1.1. Let
and
, be two
-
metrics, where
and
are two Riemannian metrics;
and
are 1- forms. Then F is projectively related to
, if and only if the following equations, holds
where
and
are the coefficients of the covariant derivative of
with respect to
;
is a scalar function and
is a 1-form on M.
Corollary 1.1. Let
and
, be two
-
metrics, where
and
are two Riemannian metrics;
and
are 1- forms. Then F is projectively flat if the following relation holds:
(1.3)
where
and
are the coefficients of the covariant derivative of
with respect to
;
is a scalar function and
is a 1-form on M.
Theorem 1.2. Let
the
-metric an
n-dimensional manifold M, with
is a Riemannian metric;
is a 1-form. Then F is locally projectively flat if and only if
(1.4)
Finally, we have shown that the generalized
-metric satisfy the sign property.
2. Preliminaries
Definition 2.1. [1] Let
(2.1)
where
are the spray coefficients of F. The tensor
is called the Douglas tensor. If Douglas tensor vanishes then Finsler metric is called Douglas metric.
Some interesting results concerning Douglas metrics are recently obtained in [10] & [11].
The function
is a
positive function on an open interval
and it satisfies the following condition:
(2.2)
Also, F is a Finsler metric if and only if
for any
.
In general, the
-metrics are defined as follows:
Definition 2.2. [1] For a given Riemannian metric
and one form
, satisfying
for
, then:
,
, is called
-metric.
The covariant derivative of
with respect to
is
. Also, in [1], the following notations are given:
(2.3)
It is clear that
if and only if
is closed. Also, we can take:
If we consider the fundamental tensor of Randers space
, then we have the following formulae
The geodesic coefficients
of F and the geodesic coefficients
of
, are related as follows (see [1]):
(2.4)
where
(2.5)
In [2] and [4], the condition for an
-metric to be locally projectively flat is presented as follows:
Lemma 2.1. A Finsler space
is locally projectively flat if and only if
(2.6)
In [12], we have the following condition for an
-metric to be a Douglas metric
(2.7)
where
and
.
Theorem 2.3. [12] Let
be an
-metric on an open
subset
, where
and one form
. Let
. Suppose that the following conditions holds
a)
is not parallel with respect to
;
b) F is not of Randers type;
c)
everywhere or
on U. Then F is a Douglas metric on U if and only if the function
satisfies the following ODE
(2.8)
and the covariant derivative
of
with respect to
satisfies the following equation
(2.9)
where
is a scalar function on U and
are constants with
.
Remark: The above equation holds good in dimension
.
3. Main Results
By the Theorem 2.1, we compute the coefficients
for
,
taking into account that
, where
, using Equation (2.9), we get
(3.1)
Next, we obtain
(3.2)
Make use of (2.5) for
, we get
(3.3)
Plugging (3.3) in (2.4), we get
(3.4)
where
is given in (3.2).
Now, we can formulate the first result:
Remark. The
-metric
is a Douglas metric with respect to Theorem 2.1, if and only if (3.1) is of the form
for some scalar function
, where
represents the coefficients of the covariant derivative
with respect to
. In this case
is closed.
If
is closed, then
and
.
Replace (3.2) in (3.4), we get:
(3.5)
We consider a scalar function
on
, i.e.,
(3.6)
From (3.5) and (3.6), we get
(3.7)
Since RHS of above equation is in quadratic form, thus there must be a 1-form
, such that
Then, we get
(3.8)
Using (3.1) and (3.8) and also the above remark, we can conclude the following result
Theorem 3.4. Let
and
, be two
-
metrics, where
and
are two Riemannian metrics;
and
are 1- forms. Then F is projectively related to
, if and only if the following equations, holds
where
and
are the coefficients of the covariant derivative of
with respect to
;
is a scalar function and
is a 1-form on M.
The proof is obtained using (3.1) and (3.8). Also, we can now formulate the following corollary:
Corollary 3.2. Let
and
, be two
-
metrics, where
and
are two Riemannian metrics;
and
are 1- forms. Then F is projectively flat if the following relation holds:
(3.9)
where
and
are the coefficients of the covariant derivative of
with respect to
;
is a scalar function and
is a 1-form on M.
Theorem 3.5. Let
the
-metric an
n-dimensional manifold M, with
is a Riemannian metric;
is a 1-form. Then F is locally projectively flat if and only if
(3.10)
Proof: We apply lemma 1.1, using
First, we compute
(3.11)
Then, we obtain
(3.12)
From (3.11), replacing k and i and substituting
, we get
(3.13)
Finally, substituting (3.12) and (3.13) in (2.6), we obtain
(3.14)
Thus
This completes the proof of necessity. The converse part follow easily.
Theorem 3.6. Let
the
-metric given by (1.1), be locally projectively flat. Assume that
is locally projectively flat. Then
(3.15)
where
Since
is locally projectively flat and from (2.6), we get
(3.16)
From (3.10) and (3.16), we get
(3.17)
Use definitions of P and Q and dividing with
in (3.17), we get
Hence the proof.
From [13], we have the following:
Definition 3.3. We say that an
-metric
on a manifold M, satisfy the sign property, if the function
has a fix sign on a symmetric interval
. Here, with s is denoted
.
Let us consider the metric (1.1),
, with
.
In this case, we have:
We conclude that, for
,
has a fix sign.
Thus metric (1.1) satisfy the sign property.
4. Conclusion
In this paper, we have obtained some important results concerning the projective change and locally projective flatness of the generalized
-metric
(
, 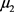 and
and  are constants). Further, we have
are constants). Further, we have
shown that the generalized 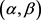 -metric satisfy the sign property.
-metric satisfy the sign property.
Acknowledgements
The authors express their sincere thanks to the reviewer for his valuable comments that greatly improved the manuscript.